Properties of Whole Numbers For Multiplication
Multiplication Properties of Whole Numbers
Understanding the properties of whole numbers in multiplication is fundamental for grasping more complex mathematical concepts later on.
What are whole numbers?
Whole numbers are a set of numbers, including all natural numbers and zero.
Example: Set of Whole numbers
W = {0, 1, 2, 3, 4, 5, 6, 7, 8, 9…… }
If we add add zero in the set of natural numbers, we get a set of whole numbers. it is denoted by W.
W = {0, 1, 2, 3, 4, 5,…}
There are six properties of multiplication of whole numbers.
These properties are help us to solve the problems easily.
1. Closure Property of Whole Numbers:
If we multiply two whole numbers, we get a whole number.
a and b are two whole numbers, then their product a x b is also a whole number.
let’s take examples of pairs of whole numbers and multiply them;
Example: (i) 7 x 8 = 56
We note that the product is always a whole number.
Imagine you have an array of dots. One array has 3 rows and 4 columns, and the other has 4 rows and 3 columns. Both arrays have the same total number of dots.
Therefore, whole numbers are closed under multiplication .
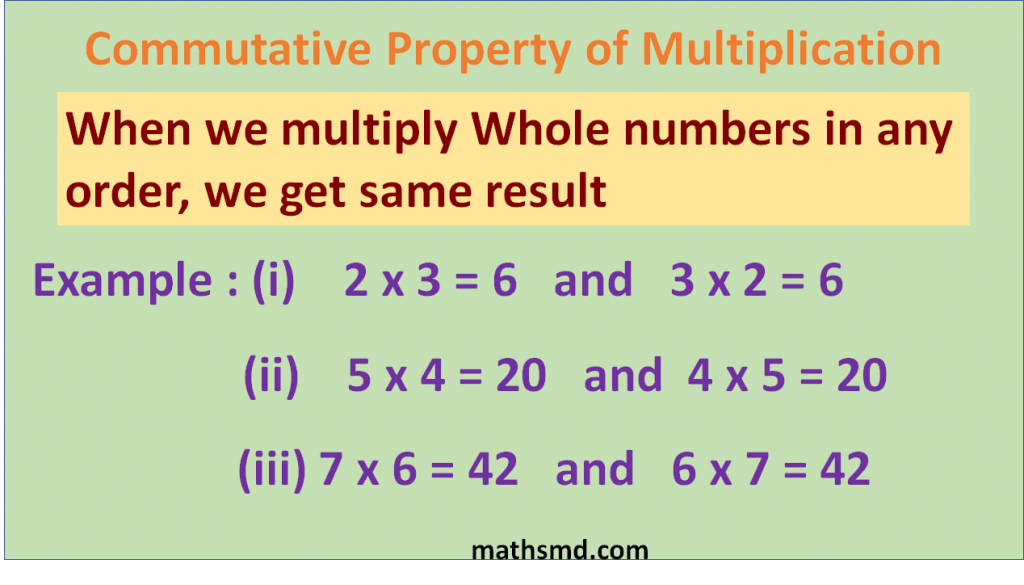
2. Commutative Property of Whole Numbers
The commutative property of multiplication states that the order in which two numbers are multiplied does not change the product. In other words:
a×b=b×a
For example:
3×4=4×3=12
This property holds true for any whole numbers.
3. Associative Property of Whole Numbers:
The associative property of multiplication states that the way in which numbers are grouped in multiplication does not change their product.
In other words:
(a×b)×c=a×(b×c)
For example:
(2×3)×4=2×(3×4)
= 6×4=2×12
24=24
Grouping the numbers differently doesn’t affect the product.
If you have three boxes and each box contains 4 sets of 3 balls, it’s the same as if you have 2 sets of boxes with 12 balls each.
This property shows that no matter how you group the numbers, the product remains the same.
4. Identity Property of Whole Numbers:
The identity property of multiplication states that any number multiplied by 1 remains unchanged. In other words:
a×1=a
For example:
7×1=7
If you have 7 apples and you multiply them by 1, you still have 7 apples.
This property is crucial because it identifies 1 as the “multiplicative identity.”
5. Distributive Property of Whole Numbers:
The distributive property combines addition and multiplication. It states that multiplying a number by a sum of numbers is the same as multiplying each addend individually by the number and then adding the products. In other words:
a×(b+c)=(a×b)+(a×c)
For example:
2×(3+4)=(2×3)+(2×4)
2×7=14
6+8=14
you have 2 groups, each with (3 + 4) objects. You can distribute the multiplication as 2 groups of 3 objects plus 2 groups of 4 objects.
This property is particularly useful in simplifying algebraic expressions.
6. Zero Property of Whole Numbers:
The zero property of multiplication states that any number multiplied by zero is zero.
In other words:
a×0=0
For example:
9×0=0
If you have 9 objects and you multiply them by zero, you have zero objects left.
This property is important because it shows that zero multiplied by any number always results in zero.
Putting It All Together
These properties help simplify multiplication and make calculations easier. They are foundational for understanding algebra, number theory, and more advanced mathematical concepts.
Here are a few example problems illustrating these properties:
Example 1: Commutative Property
5×6=6×5=30
Example 2: Associative Property
(3×4)×2=3×(4×2)
12×2=3×8
=24
Example 3: Identity Property
8×1=8
Example 4: Distributive Property
3×(5+2)=(3×5)+(3×2)
3×7 = 15+6 = 21
Example 5: Zero Property
4×0=0
By understanding and applying these properties, students can perform multiplication more efficiently and accurately.