Commutative Property of Rational Numbers
Commutative Property of Rational Numbers
Commutative Property
The commutative property of rational numbers states that when any two rational numbers are added or multiplied in any order the result we get same result.
In case of subtraction and division, if order of numbers is change the result will also change.
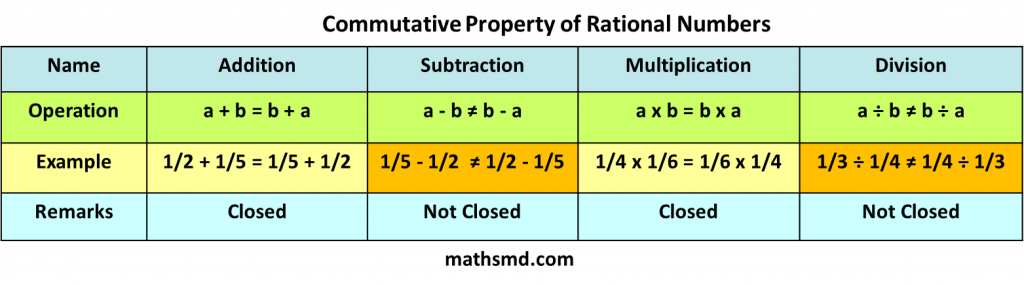
We will understand these properties on each operation with examples.
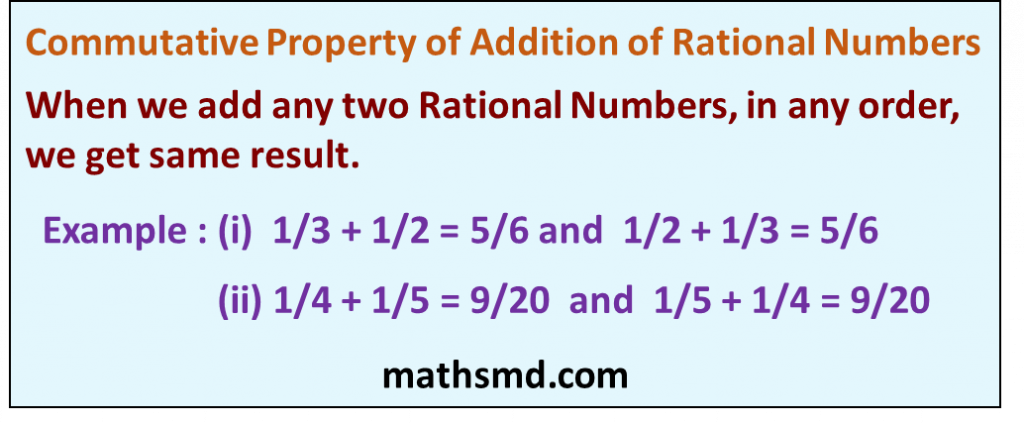
Commutative Property for Addition
Commutative property for addition of rational numbers states that any two rational numbers are added in any order the result does not change.
i.e., if a and b are two rational numbers then,
a + b = b + a is also a rational number.
Example: Solve 1/3 + 1/5
= (5 + 3)/15
= 8/15
= 1/5 + 1/3
= (3 + 5)/15
= 8/15
1/3 + 1/5 = 1/5 + 1/3
8/15 = 8/15
We can say that The sum of two rational numbers does not depend on the order in which they are added, i.e., a and b are rational numbers,
a + b = b + a
Here result is 8/15, which is also a rational number.
That is for any two rational numbers a and b, a + b is also a rational number.
We can say that the rational numbers are commutative under addition.
Commutative Property for Subtraction
Subtraction is not commutative for rational numbers.
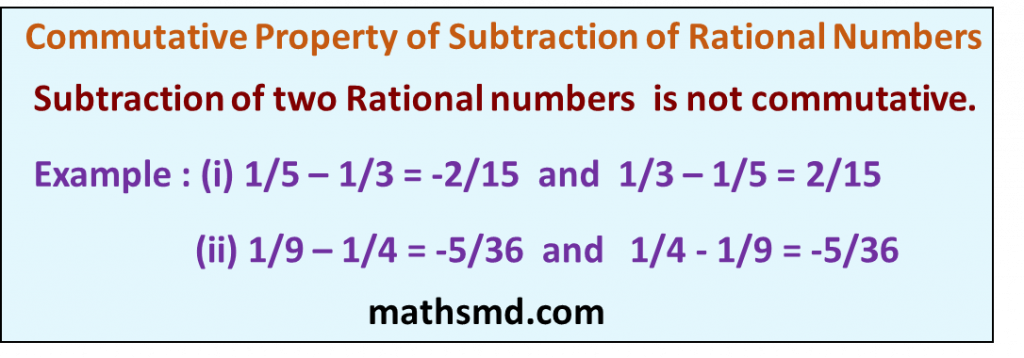
Let us consider a/b and c/d, be two rational numbers then,
a/b – c/d ≠ c/d – a/b
Example: 1/2 – 1/3
= (3 – 2)/6
= 1/6, whereas
1/3 – 1/2
= (2 – 3)/6
= -1/6
1/2 – 1/3 ≠ 1/3 – 1/2
This means subtraction is not commutative for rational numbers.
That is for any two rational numbers a and b,
a – b ≠ b – a
Commutative Property for Multiplication
Commutative property for multiplication of rational numbers states that when any two numbers are multiplied in any order it does not change the result.
i.e., if a and b are rational numbers,
a x b = b x a is also a rational number.

Example: 4/5 x 2/3
4/5 x 2/3 = 8/15
2/3 x 4/5 = 8/15
∴ a x b = b x a
4/5 x 2/3 = 2/3 x 4/5 = 8/15
Here result is 8/15, which is also a rational number.
We can say that the rational numbers are commutative under multiplication.
Commutative Property for Division
Division is not commutative for rational numbers.
a ÷ b ≠ b ÷ a

Example: 2/5 ÷ 4/3 and 4/3 ÷ 2/5
2/5 ÷ 4/3 = 6/20 and
4/3 ÷ 2/5 = 20/6, whereas
2/5 ÷ 4/3 ≠ 4/3 ÷ 2/5
We can see that the expression on both sides are not equal.
The result in both cases are different, 6/20 and 20/6.
This means for any two rational numbers a and b.
a ÷ b ≠ b ÷ a,
So division is not commutative for rational numbers.