Dividing Fractions by a Mixed Fraction With Examples

The steps dividing fractions by a mixed fraction are almost same to dividing fractions by a fraction.

How to divide fractions by mixed Fraction

Let us see the steps to divide a given fraction by a mixed fraction with an example.

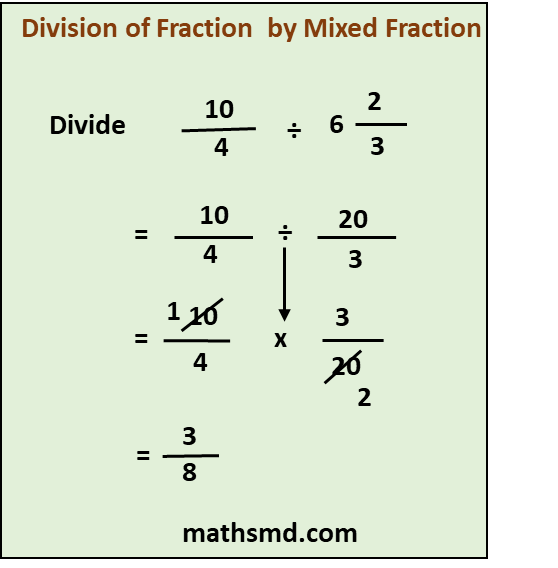
Example: Divide 3/2 ÷ 2 1/4

Step 1: Convert the given mixed fraction into improper fraction.

We have learned how to convert mixed fractions to improper fractions.

Here, 2 is a whole number and 1/4 is a proper fraction.

So, we change mixed fraction 2 1/4 into improper fraction i.e.,

[(4 x 2) + 1]/ = 9/4

2 1/4 is same as 9/4.

Now our problem is 3/2 ÷ 9/4

Step2: Multiply by the reciprocal of the divisor.

= 3/2 x 4/9

= (3 x 4)/(2 x 9)

= 12/18

Step 3: Simplify/reduce if needed/possible.

= 2/3

Therefore, 3/2 ÷ 2 1/4 = 2/3

Example: Divide 2/5 ÷ 3 1/5

Change mixed fraction into improper fraction.

3 1/5 = [(5 x 3) + 1]/5

= (15 + 1)/5

= 16/5

Now our problem is 2/5 ÷ 16/5

= 2/5 ÷ 16/5

Multiply by the reciprocal of the divisor.

= (2/5 x 5/16)

= (2 x 5)/(5 x 16)

= 10/80

Simplify/reduce if needed/possible.

∴ 10/80 = 1/8

Therefore, 2/5 ÷ 3 1/5 = 1/8

Example: Divide 7/3 ÷ 4 1/5

Change mixed fraction into improper fraction.

4 1/5 = [(5 x 4) + 1]/5

= (20 + 1)/5

= 21/5

Now our problem is 7/3 ÷ 21/5

= 7/3 ÷ 21/5

Multiply by the reciprocal of the divisor.

= (7/3 x 5/21)

= (7 x 5)/(3 x 21)

= (7 x 5)/(3 x 21)

Simplify/reduce if needed/possible.

∴ (7 x 5)/(3 x 21) = 5/(3 x 3)

= 5/9

Therefore, 7/3 ÷ 4 1/5 = 5/9

Example: Divide 3/5 ÷ 2 6/10

Change mixed fraction into improper fraction.

2 6/10 = [(10 x 2) + 6]/10

= (20 + 6)/10

= 26/10 Now our problem is 3/5 ÷ 26/10

= 3/5 ÷ 26/10

Multiply by the reciprocal of the divisor.

= (3/5 x 10/26)

= (3 x 10)/(5 x 26)

Simplify/reduce if needed/possible.

= (3 x 2)/(1 x 26)

∴ (3 x 1)/(1 x 13) = 3/(13)

= 3/13

Therefore, 3/5 ÷ 2 6/10 = 3/13