Fractions on Number Line Representation – Examples

What is a Fraction?

A fraction represents a part of a whole. It consists of a numerator (top number) and a denominator (bottom number). For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator. This fraction means 3 parts out of 4 equal parts.

Number Line Basics

A number line is a straight line with representing numbers visually. A number line can be extended infinitely in any direction. A number line usually represent horizontally. If we move from left to right the numbers on number line increase and move from right to left numbers are decrease.

In a number line numbers placed at equal intervals along its length.

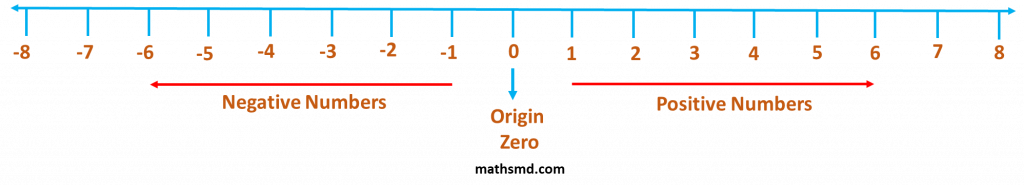
To show fractions on a number line is very helpful to represent how a whole number is divided equally into parts.

It helps in visualizing numbers and their relationships. For fractions, the number line is divided into equal segments based on the denominator.

Fractions on Number Line: Representing fractions on a number line, is similar to plotting whole numbers and integers. fractions represent parts of a whole.

How to Plot Fractions on Number Line

Understanding fractions on a number line can be made easier with clear diagrams and examples.

Here’s a step-by-step guide with diagrams and examples.

Understanding Fractions on a Number Line

Steps to Represent Fractions on a Number Line

1. Draw a Number Line:
   • Draw a horizontal line and mark the whole numbers, starting from 0.
2. Divide into Equal Parts:
   • To represent fractions, divide the segment between each whole number into equal parts based on the denominator.
3. Mark the Fractions:
   • Count the segments from 0 to locate the fraction on the number line.

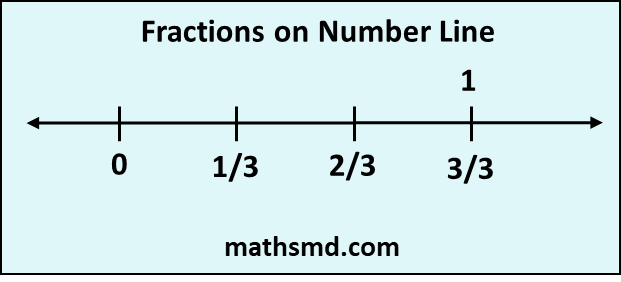
Example 1: Placing 3/3 on a Number Line

1. Draw the number line and mark 0 and 1.
2. Divide the segment between 0 and 1 into 3 equal parts (since the denominator is 3).
3. Count 3 parts from 0 to locate 3/3.
4. Here, 1 the represents 3/3.

Example 2: Plotting 1/2​

1. Draw the Number Line:
   • Start with a line from 0 to 1.
2. Divide the Segment:
   • Since the denominator is 2, divide the segment between 0 and 1 into 2 equal parts.
3. Mark the Fraction:
   • 1/2​ is one part out of the two, so mark it halfway between 0 and 1.

Diagram:

0    1/2    1
|-----|-----|
0    0.5    1

Example 3: Plotting 3/4​

1. Draw the Number Line:
   • Start with a line from 0 to 1.
2. Divide the Segment:
   • Since the denominator is 4, divide the segment between 0 and 1 into 4 equal parts.
3. Mark the Fraction:
   • 3/4​ is three parts out of the four, so mark it three-quarters of the way from 0 to 1.

Diagram:

0   1/4   1/2   3/4   1
|----|----|----|----|
0   0.25  0.5  0.75  1

Example 4: Plotting 5/3​

1. Draw the Number Line:
   • Start with a line from 0 to 2 (since 5/3 is more than 1).
2. Divide the Segment:
   • Since the denominator is 3, divide each segment between whole numbers (0 to 1 and 1 to 2) into 3 equal parts.
3. Mark the Fraction:
   • 5/3​ is five parts out of the three, which extends beyond 1. So, it is 1 whole and 2/3.
   • Mark the point 2/3 past 1.

Diagram:

1/3   2/3   1   4/3   5/3   2
|----|----|----|----|----|----|
0   0.33  0.67  1   1.33  1.67  2

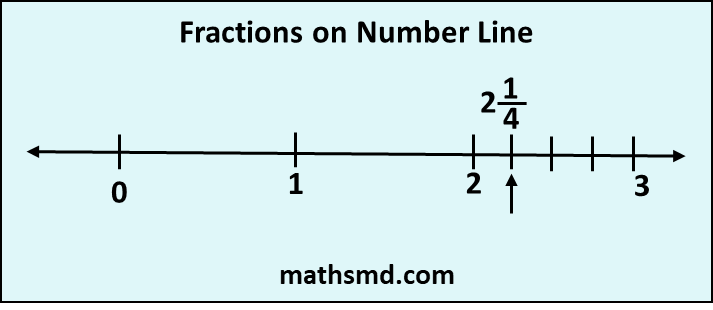
Example 5: Plotting 9/4​

1. Draw the Number Line:
   • Start with a line from 0 to 3 (since 9/4 is more than 2).
2. Divide the Segment:
   • Since the denominator is 4, divide each segment between whole numbers (0 to 1, 1 to 2 and 2 to 3) into 4 equal parts.
3. Mark the Fraction:
   • 9/4​ is nine parts out of the four, which extends beyond 2. So, it is 2 whole and 1/4.
   • Mark the point 1/4 past 2.

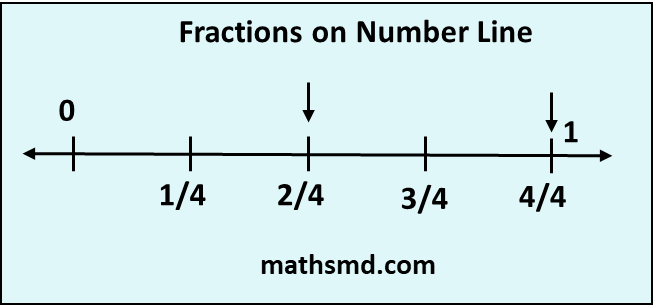
Example 6: Plotting 2/4​ and 4/4

Draw a horizontal line with arrows on both ends. This line represents the number line.

• Identify Whole Numbers: Mark the whole numbers on the number line. let’s mark from 0 to 1.
• Divide the Segment: To place fractions, divide the segment between 0 and 1 into 4 equal parts based on the Denominator.
• Mark the Fractions: Count 2 and 4 parts from 0 to locate 2/4 and 4/4. 2/4​ is two part out of the four and 4/4 is 1, so mark it two-quarters of the way from 0 to 1 and mark 1.

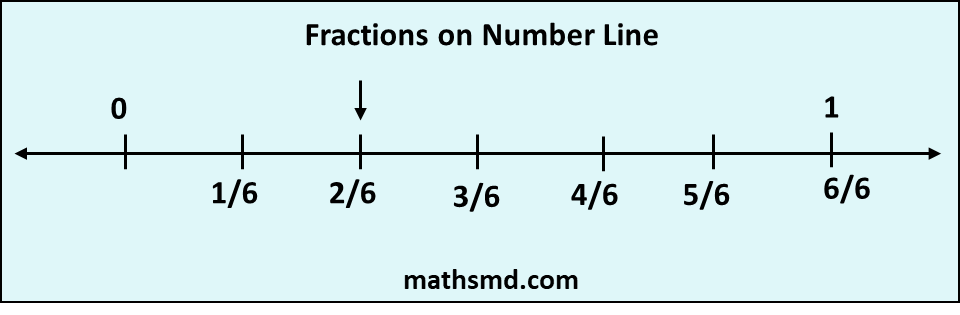
Example 7: Placing 2/6​ on a Number Line

1. Draw the number line and mark 0 and 1.
2. Divide the segment between 0 and 1 into 6 equal parts (since the denominator is 6).
3. Count 2 parts from 0 to locate 2/6.
4. Here, the represents 2/6​.

Practice Exercise:

1. Draw a number line from 0 to 2.
2. Place 1/2​, 3/2​, and 5/4​ on the number line.

Negative Fractions on a Number Line

Explaining negative fractions on a number line can be made clear and engaging with detailed examples and diagrams.

Here’s a step-by-step guide:

Step-by-Step Explanation

1. Understanding the Number Line:
   • The number line is a straight line with numbers placed at intervals. It usually has zero in the middle, positive numbers to the right, and negative numbers to the left.
2. Introducing Fractions:
   • Fractions represent parts of a whole. For example, 1/2 is one half, 3/4 is three quarters, etc.
3. Positive and Negative Fractions:
   • Positive fractions are to the right of zero.
   • Negative fractions are to the left of zero.

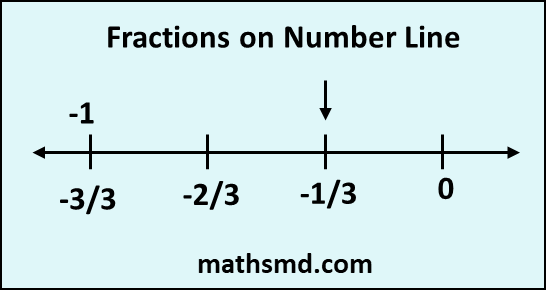
Diagram and Examples

1. Drawing the Number Line:

Draw a horizontal line and mark the center as 0. Add a few positive and negative integers equally spaced on both sides of 0.

<----|----|----|----|----|----|----|----|----|----|---->

-3 -2 -1 0 1 2 3

2. Placing Fractions on the Number Line:

To place fractions, we divide the spaces between integers into equal parts. Let’s place the fractions -1/2, -1/4, and -3/4 on the number line.

• Dividing into Fourths:
  • Each space between the integers is divided into 4 equal parts for fourths.

3. Plotting Negative Fractions:

Example 1: Plot -1/2

• Locate -1 and 0.
• Divide the space between -1 and 0 into 2 equal parts.
• -1/2 is halfway between -1 and 0.

Example 2: Plot -1/4

• Locate -1 and 0.
• Divide the space between -1 and 0 into 4 equal parts.
• -1/4 is the first part from 0 towards -1.

Example 3: Plot -3/4

• Locate -1 and 0.
• Divide the space between -1 and 0 into 4 equal parts.
• -3/4 is the third part from 0 towards -1.

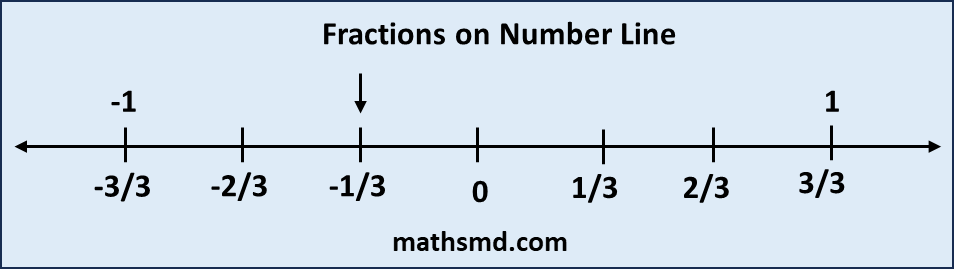
Detailed Diagram:

<----|----|----|----|----|----|----|----|----|----|---->
   -1   -3/4  -1/2  -1/4   0    1/4   1/2   3/4   1

1. -1/2 is halfway between -1 and 0.
2. -1/4 is one-fourth of the way from 0 to -1.
3. -3/4 is three-fourths of the way from 0 to -1.

<----|----|----|----|----|----|----|----|----|----|---->
 -1   -3/4  -1/2  -1/4   0    1/4   1/2   3/4   1

By practicing with different fractions, students will gain a better understanding of how fractions fit on a number line.

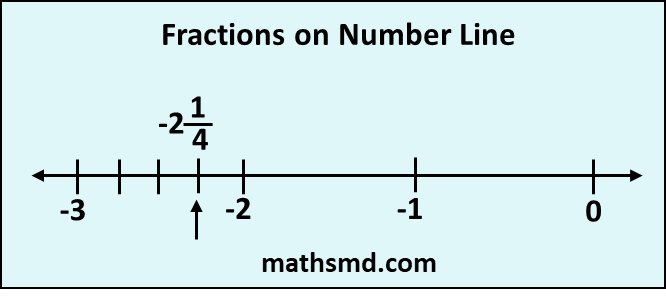
Tips

• Use Visual Aids: Draw large number lines and use colored markers for different fractions.
• Interactive Activities: Have students place fractions on a number line using sticky notes.
• Relate to Real Life: Use examples like slicing a pizza or dividing chocolate bars to make fractions relatable.

Key Points to Remember:

• The denominator tells you into how many equal parts the segment between 0 and 1 is divided.
• The numerator tells you how many of those parts you need to count from 0.
• Fractions greater than 1 can also be represented by extending the number line beyond 1 and repeating the same steps.