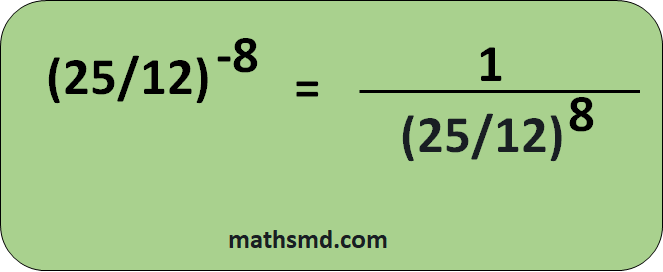Negative Exponents

In this tutorial, we will learn about negative exponents.

When we change a negative exponent into positive exponent, we write the reciprocal of the given positive exponent. Negative sign means the reciprocal of the given number.

For any non zero number a, (a^-m = 1/a^m).

Where m is a positive integer and a^-m is the multiplicative inverse of a^m.

Writing Negative exponents as Positive exponents

Lets understand how to change the negative exponents into positive exponents.

Example: (2^-3)

Start with (2^-3) to multiply by the number 2³ and divided by 2³.

That’s equal to 2^-3 (multiply by 2³ and divide by 2³)

In short we multiply the number 2^-3 by 1.

(2³/2³ = 1), so the original number is not change.

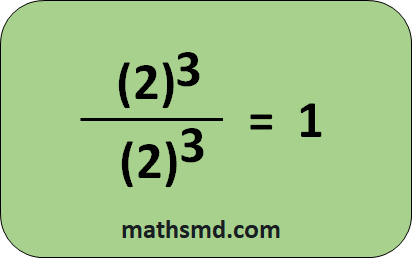
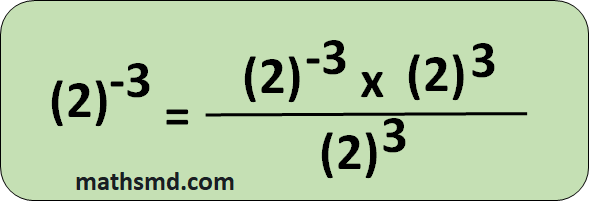
We can use the multiplication law to write down as,

We write the numerator as 2 raised to (-3 + 3)

(-3 + 3 = 0),

2º divided by 2³,

(Any non zero number)º = 1

So write 2^-3, as 1/2³

This is our number (2^-3), and answer is 1 is divided by 2³. so all we did is change the sign of exponent and shifted below 1.

If a = 0, we have 0 in denominator, it is indeterminate.