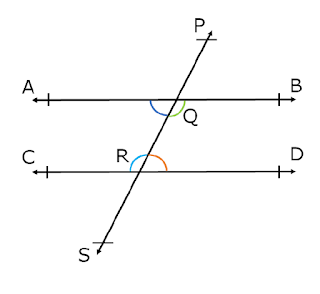
Theorem – Same side of the transversal, Angles are Supplementary

If a transversal intersect two parallel lines, then each pair of interior angles on the Same side of the transversal are Supplementary.

Given: Two parallel Lines AB and CD, and PS be transversal intersecting AB at Q and CD at R.

To Prove: Sum of interior angles on the Same side of the transversal is Supplementary.

i.e.  ∠AQR + ∠CRQ = 1800 and ∠BQR + ∠DRQ = 1800

Proof: Parallel lines AB and CD, and PS be transversal
intersecting AB at Q and CD at R.

So that, ∠AQP = ∠CRQ (Corresponding angles)…1

For line PS 

∠AQP + ∠AQR = 1800  (Linear pair)….2

Putting the value of (1) in (2)

∠AQR + ∠CRQ = 1800

Similarly we can prove that

∠BQR + ∠DRQ = 1800

Therefore, sum of interior angles on same side of transversal is 1800 .

                                           Hence proved.

Related posts:

  1. The sum of the angles of a triangle is 180-Theorem
  2. Theorem – Alternate Interior Angles are Equal
  3. Theorem – If the diagonals of a Quadrilateral bisect each other then that Quadrilateral is a Parallelogram
  4. Lines are parallel to a third line then the lines are parallel to each other.
  5. Theorem – Alternate interior angles is equal, then the two lines are parallel
  6. The angle subtended by an arc at the centre is double of the angle subtended by it at any point on the remaining part of the circle – Theorem – geometry
  7. Prove that the angle in a semicircle is a right angle – Theorem – Geometry
  8. Theorem – The line segment joining the mid-points of two sides of a triangle is parallel to the third side.
  9. Exterior Angle of a Triangle – Theorem
  10. Theorem – A diagonal of a parallelogram divides it into two congruent triangles

Leave a Reply

Your email address will not be published. Required fields are marked *