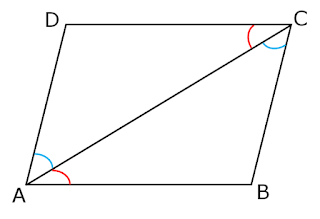
Theorem – A diagonal of a parallelogram divides it into two congruent triangles
A diagonal of a parallelogram, divides it into two congruent triangles.
Given: A parallelogram ABCD and AC is a diagonal, the diagonal AC divides parallelogram ABCD into two triangles △ ABC and △ CDA.
To prove: These triangles △ ABD and △CDA are congruent,
△ ABC ≅ △ CDA
Proof: In △ ABC and △ CDA
BC ∥ AD and AC is a transversal.
So, ∠BCA = ∠DAC (pair of alternate angles)
Similarly, AB ∥ DC and AC is a transversal.
So, ∠BAC = ∠DCA (pair of alternate angles)
and AC = CA (Common)
So, △ ABC ≅ △CDA (By ASA rule)
or diagonal AC divides parallelogram ABCD into two congruent triangles △ ABC and △ CDA.
Hence proved.