Circle – Area – Formula and Problems with Solutions

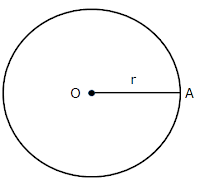
 Area of a circle is A = 𝝿r²

= 𝝿 (pi) x (radius)² , where r is radius of the circle.

or when we know the diameter A = (𝝿/4) x D²

when we know circumference A = c²/4𝝿

Let us now solve problems on area of a circle.

Here are examples demonstrating the area of a circle with different radii.

Example 1: Small Circle

Radius (r): 3 cm

Area Calculation: A=πr²

A=π(3 cm)²

A=3.14×9 cm²A = 3.14

A=28.26cm²

1. Draw a circle with a radius of 3 cm.
2. Show the center (O) and the radius (r).

Example 2: Medium Circle

Radius (r): 5 cm

Area Calculation: A=πr²

A=π(5cm)²

A=3.14×25 cm²

A=78.5cm²

1. Draw a circle with a radius of 5 cm.
2. Show the center (O) and the radius (r).

Example 3: Medium Circle

The radius of a circle is 7 cm, what is the area of the circle.

Solution : Area of a circle is A = 𝝿r²
here radius of circle is 7 cm.
                   so, area of circle = 𝝿 x 7²
                                            = 3.14 x 7 x 7 cm²
               Area of a circle is A = 153.86 cm²

Example 4: Large Circle

Radius (r): 8 cm

Area Calculation: A=πr²

A=π(8 cm)²

A=3.14×64 cm²

A=200.96cm²

1. Draw a circle with a radius of 8 cm.
2. Show the center (O) and the radius (r).

Summary of Areas:

1. Circle with radius 3 cm: Area = 28.26 cm²
2. Circle with radius 5 cm: Area = 78.5 cm²
3. Circle with radius 8 cm: Area = 200.96 cm²

Example 5:

The radius of a circle is 30 cm, what is the area of the circle.

Solution : Area of a circle is A = 𝝿r²
here radius of circle is 30 cm.
                   so, area of circle = 𝝿 x 30²
                                            = 3.14 x 30 x 30 cm²
               Area of a circle is A = 2826 cm²

Example 6:

Diameter of a circle is 9.8 cm, what is the area of the circle.

Solution : Diameter of a circle is 9.8 cm

Therefore, radius of circle = 9.8/2 cm
                                      = 4.9 cm

             Area of a circle is A = 𝝿r²
here radius of circle is 4.9 cm.
                   so, area of circle = 𝝿 x (4.9)²
                                           = 3.14 x 4.9 x 4.9 cm²
               Area of a circle is A = 75.46 cm²

Example 7:

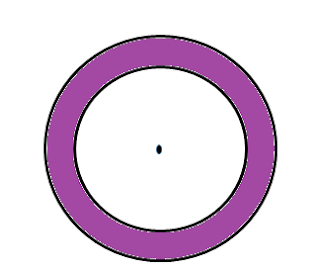
In the figure two circles with same centre. The  radius of larger circle is 8 cm, and radius of smaller circle is 4 cm.

(i)what is the area of the larger circle.
(ii)what is the area of the smaller circle.(iii)what is the area of the shaded part between two circles.

 Solution : Area of a circle is A = 𝝿r²
here radius of larger circle is 8 cm.
           so, area of larger circle = 𝝿 x 8²
                                            = 3.14 x 8 x 8 cm²     (i)    Area of larger circle is = 200.96 cm²


now, radius of smaller circle is 4 cm.
           so, area of smaller circle = 𝝿 x 4²
                                            = 3.14 x 4 x 4 cm²    (ii)    Area of larger circle is = 50.24 cm²

(iii)The area of the shaded part between two circles
                               = 200.96 – 50.24 cm^{2
  
  Area of shaded part  = 150.72} cm²

Example 8:

Find the perimeter of the given figure, which is a semicircle including its diameter.

 Solution : Diameter of given semicircle = 14 cm(5)

                           so, radius = 14/2
                                          = 7 cm
According to the question

perimeter of figure = circumference of semicircle +   diameter
                            = 𝝿r + D
                            = 22/7 x 7 + 14
                            = 22 + 14
perimeter of figure = 36 cm

By comparing these examples and their diagrams, we can see how the area of a circle increases with the radius. These visual aids help solidify the concept and make the math more engaging and understandable.