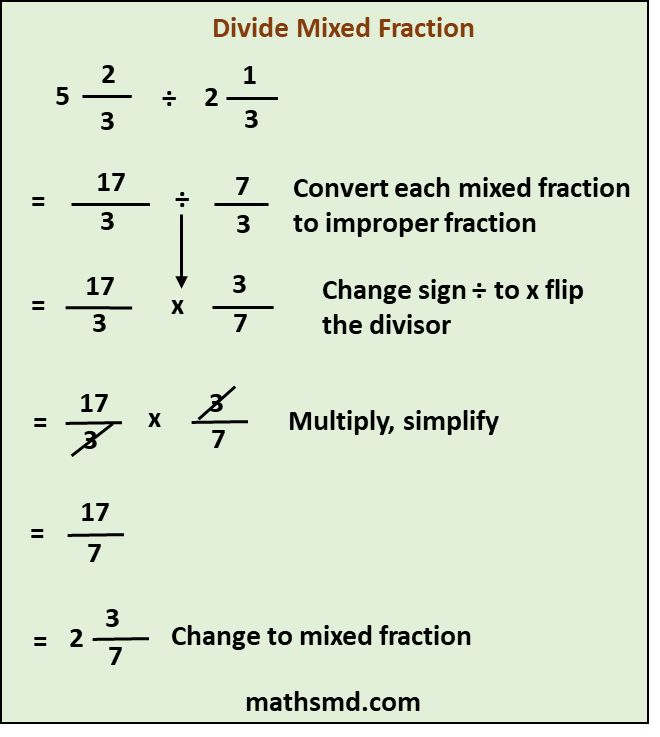
Dividing Mixed Fractions With Examples

Let us see the steps to divide a given mixed fraction by a mixed fraction with an example.

Example: Divide 3 2/4 ÷ 4 1/5

Step 1: Convert each mixed fraction into improper fraction.

3 2/4 ÷ 4 1/5

3 2/4 = 14/4 and 4 1/5 = 21/5

Now problem is 3 2/4 ÷ 4 1/5 = 14/4 ÷ 21/5

Step 2: Change the sign of (÷ to x) division to multiplication.

14/4 x 5/21

Flip(Turn) the second fraction (improper fraction), we want to divide by upside down (now a reciprocal).

reciprocal of 21/5 is 5/21

Step 3: Now multiply numerators and denominators of first fraction and second fraction separately.

(14 x 5)/(4 x 21) = 70/84

Step 4: Simplify the fractions (if needed).

Both numerator and denominator have common factors 7 and 2, therefore simplify the fraction to its lowest form.

70/84= 5/6

Step 5: If the resultant fraction is improper convert it into a mixed fraction.

Here, 5/6 is proper fraction.

Therefore, 3 2/4 ÷ 4 1/5 = 70/84 = 5/6

Example: Divide 2 3/5 ÷ 2 2/4

Step 1: Convert each mixed fraction into improper fraction.

2 3/5 ÷ 2 2/4

2 3/5 = 13/5 and 2 2/4 = 10/4

Now problem is 2 3/5 ÷ 2 2/4 = 13/5 ÷ 10/4

Step 2: Change the sign of (÷ to x) division to multiplication.

13/5 x 4/10

Flip(Turn) the second fraction (improper fraction), we want to divide by upside down (now a reciprocal).

reciprocal of 10/4 is 4/10

Step 3: Now multiply numerators and denominators of first fraction and second fraction separately.

= (13 x 4)(5 x 10) = 52/50

Step 4: Simplify the fractions (if needed).

(13 x 4)/(5 x 10) Both numerator and denominator have common factor 2, therefore simplify the fraction to its lowest form.

52/50= 26/25

Step 5: If the resultant fraction is improper fraction, so convert it into a mixed fraction.

Here, 26/25 is an improper fraction so convert it into a mixed fraction.

26/25 = 1 1/25

Therefore, 2 3/5 ÷ 2 2/4 = 26/25 = 1 1/25