What are Co-Interior Angles
Co-internal angles
Co-interior angles, also known as consecutive interior angles or same-side interior angles, are pairs of angles that lie on the same side of a transversal and inside the two lines it intersects. These angles have specific properties depending on whether the lines they intersect are parallel or not.
Definition and Identification
(1) Transversal Line:
A line that cuts across two or more (usually parallel) lines.
(2) Interior Angles: Angles that are on the inside of the two intersected lines.
When a transversal intersects two lines, it forms eight angles. The pairs of co-interior angles are the pairs of angles that are:
- On the same side of the transversal.
- Inside the two intersected line.
Properties of Co-Interior Angles
Non-Parallel Lines:
- When the two lines are not parallel, the co-interior angles do not have any specific relationship in terms of their measures.
Parallel Lines:

- When the two lines are parallel, the co-interior angles are supplementary. This means that the sum of the measures of the two co-interior angles is always 180º.
- If ∠A and ∠B are co-interior angles, then: ∠A+∠B=180
Example
Consider two parallel lines
l1 and l2 intersected by a transversal t:
Let’s label the angles formed at the intersections as follows:
In this diagram:
- ∠1 and ∠4 are co-interior angles.
- ∠2 and ∠3 are co-interior angles.
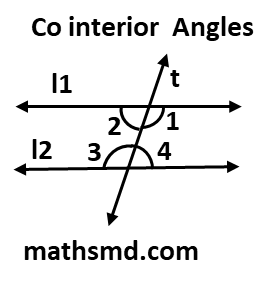
If l1and l2 are parallel, then:
∠1+∠4=180 and ∠2+∠3=180
Proof of Supplementary Property
When two parallel lines are cut by a transversal, corresponding angles are equal, and alternate interior angles are equal. This can be used to prove that co-interior angles are supplementary.
Let’s consider the same example:
- ∠1 and ∠2 are corresponding angles, so ∠1=∠2.
- ∠2 and ∠3 are alternate interior angles, so ∠2=∠3.
- Therefore, ∠1=∠3.
Since ∠3 and ∠4 form a straight line (linear pair), they are supplementary:
∠3+∠4=180
Substituting ∠1 for ∠3:
∠1+∠4=180
This proves that the co-interior angles
∠1 and ∠4 are supplementary.
Conclusion
Co-interior angles are a fundamental concept in geometry, especially when dealing with parallel lines and transversals. Understanding their properties helps in solving various geometric problems and proofs.