Natural Numbers – Definition, Examples and Properties
Natural Numbers – Definition, Examples and Properties
Introduction of Natural Numbers
In this tutorial, we will learn about natural numbers. In our daily life we use numbers everywhere around us, like for telling time, measuring the temperature, exchange the money, counting objects etc.
“Natural Numbers” are numbers that we use to count.
Natural numbers are an important part of the number system, including all positive integers from 1 to infinity. Natural numbers does not include 0 or negative numbers. Natural numbers are part of real numbers, that include only positive integers, but not negative integers, decimals, fractions and zero.
Natural Numbers Definition
Natural numbers are the part of real numbers, which are used for counting.
The set of natural numbers include only positive integers, i.e., 1, 2, 3, 4, 5, 6, 7, 8, 9…….∞.
The counting numbers (1, 2, 3, 4, ……..) are known as “Natural Numbers” or counting numbers.
1, 2, 3, 4, 5…..is the set of counting numbers.


The symbol of Natural Numbers is “N”
In 5th century, the great Indian mathematician, Arya Bhatt invented the number Zero.
Some mathematicians include zero(0) in Natural Numbers, and others start from 1.
Is 0 a Natural Number?
0 is not a natural number because we know that natural numbers are counting numbers start with 1 to infinity, and natural numbers are only positive integers. When we count anything, we start counting from 1 not from 0. 0 is a whole number and 0 has a null value.
Smallest natural Number

The smallest natural number is 1. We know that the smallest element in set of natural numbers N is 1.

Set of Natural Numbers
The symbol of natural number is ‘N’. The set of natural numbers is written as
N = {1, 2, 3, 4, 5, 6,………∞}
The set of natural numbers has 1 to infinite number of elements. It does not mean that infinite is a natural number.
Set notation it is represented as given below.
N = Set of all positive integers starting from 1.
(1) Statement Form
N = Set of all positive integers starting from 1.
(2) Roster Form
N = {11, 2, 3, 4, 5, 6, 7, 8, 9,10, 11, 12……}
(3) Builder Form
N = {x : x is an integer starting from 1}
Natural Numbers on Number Line
Below number line is represents the natural numbers.
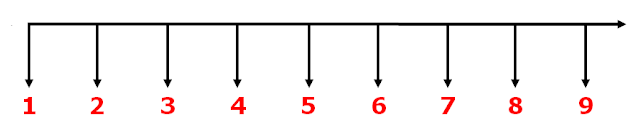
All the positive integers on the right hand side of 0, represent the natural numbers.
The natural numbers on the number line are often represented by points. The distance between these points labelled as 1 and 2 is called unit distance.
In this way go on labelling points at unit distance as 1, 2, 3, 4, 5, ..on the line.
Properties of Natural Numbers
The four operations, addition, subtraction, multiplication and division on natural numbers.
The four main properties of natural numbers is explained below in detail.
(1) Closure Property
(2) Commutative Property
(3) Associative Property
(4) Distributive Property
Let’s check all four operations in all four properties for all a, b ∈ N.
(1) Closure property

This shows that, when we add or multiply any two natural numbers the result will always be a natural number.
Subtraction and division of two natural numbers is not always a natural number.
(i) Closure Property of Addition
This shows that the sum of two natural numbers is also a natural number.

Closure property of addition, a + b = c
Where a, b and c are natural numbers.
Example: 3 + 4 = 7
Example: 15 + 9 = 24
(ii) Closure Property of Multiplication
This shows that the multiplication of two natural numbers is also a natural number.
Example: 3 x 4 = 12
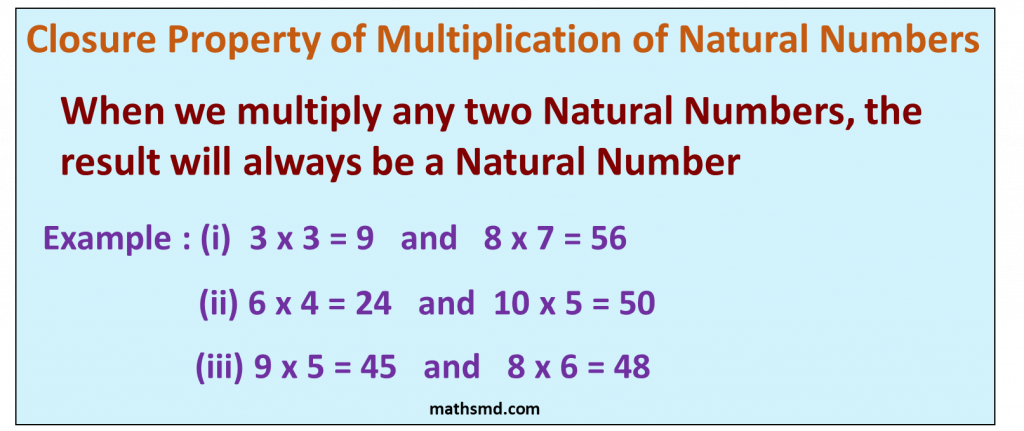
Example: 12 x 6 = 72
The product of two natural numbers is also a natural number.
Closure property of Multiplication, a x b = c
Closure property of addition a + b = c
Where a, b and c are natural numbers.
This shows that the set of natural numbers are always closed under addition and multiplication.
(iii) Closure Property of Subtraction
The Subtraction of two natural numbers is not always a natural number.
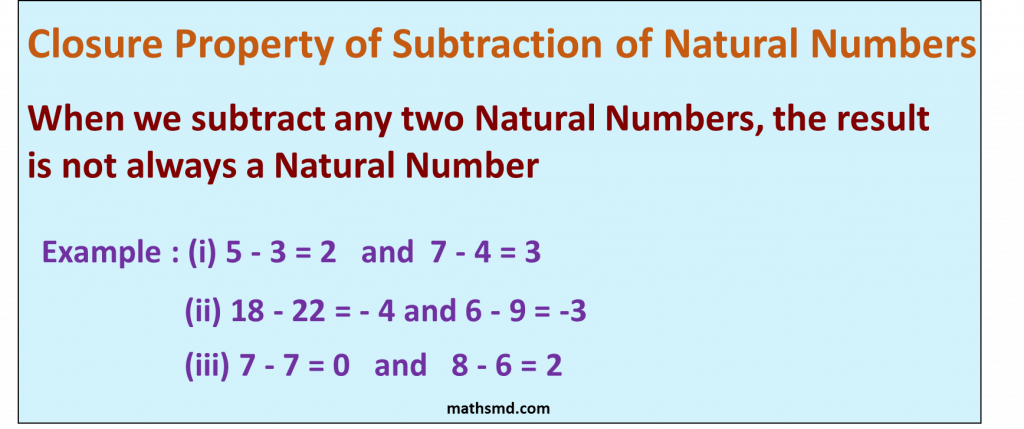
Example: 6 – 2 = 4 is a natural number, but
2 – 6 = – 4 is not a natural number.
When we subtract a larger natural number from a smaller natural number, we do not get a natural number.
(iv) Closure Property of Division
Division of two natural numbers is not always a natural number.
When we divide natural numbers the resultant number may, or may not be a natural number.

Example: 8/2 = 4 is a natural number, but
2/8 = 1/4 is not a natural number.
This shows that set of natural numbers are not closed under subtraction and division.
The addition and multiplication of two or more natural numbers will always a natural number, but this is not closed under subtraction and division. Subtraction or division of two natural numbers will not always give a natural number.
(2) Associative Property
The sum of any three natural numbers remains the same even if the grouping of numbers is changed.
(i) Associative property of Addition
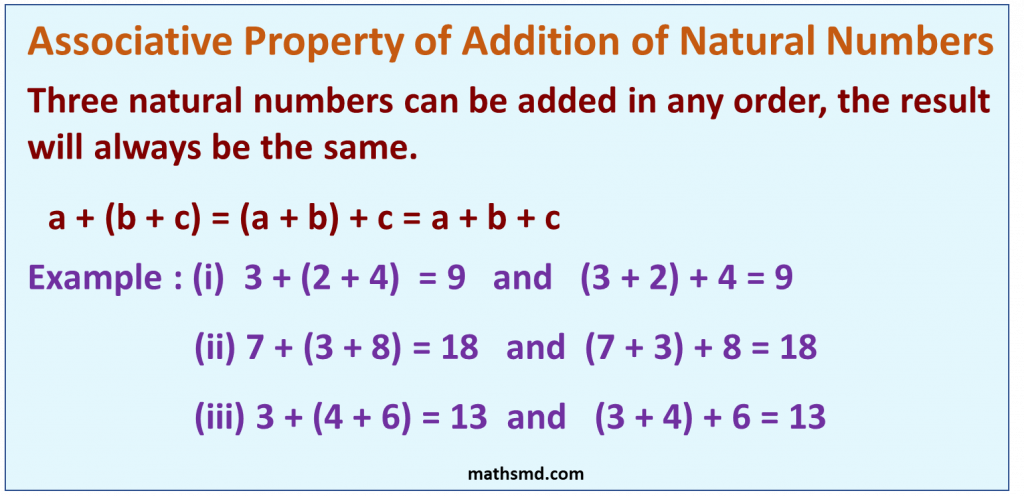
a + (b + c) = (a + b) + c
3 + (4 + 2) = (3 + 4) + 2
3 + 6 = 9
7 + 2 = 9
(ii) Associative property of Multiplication
The product of any three natural numbers remains the same even if the grouping of numbers is changed.
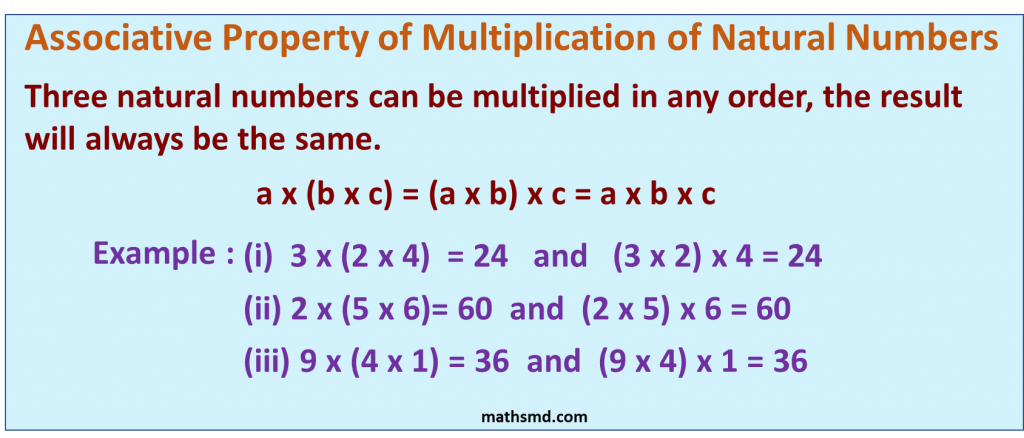
a x (b x c) = (a x b) x c
3 x (4 x 2) = (3 x 4) x 2
3 x 8 = 12 x 2 = 24

This shows that the set of natural numbers are associative under addition and multiplication.
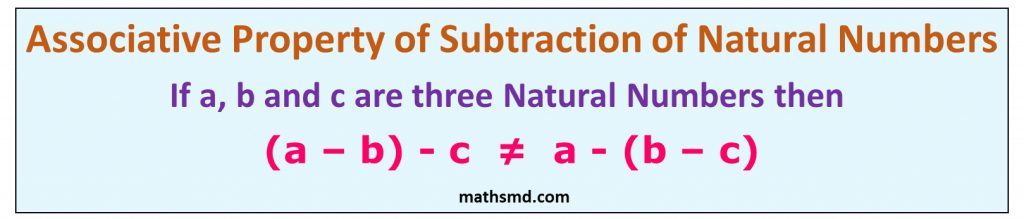
(iii) Associative property of Subtraction
In the subtraction of natural numbers, when grouping is changed the result changes. Associative property of subtraction is not true for subtraction.
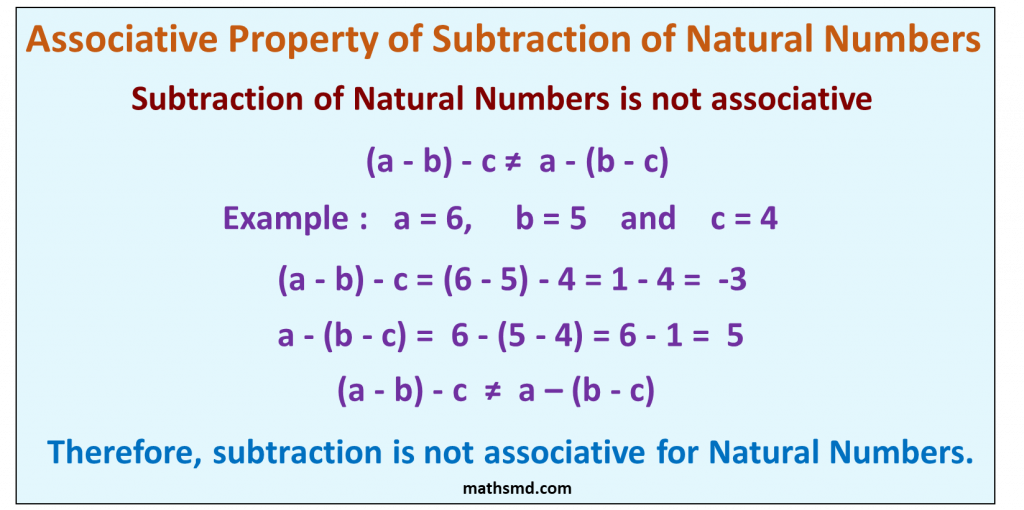
a – (b – c) ≠ (a – b) – c
Example: 9 – (7 – 2) = 9 – 5 = 4 and
(9 – 7) – 2 = 2 – 2 = 0
Example: 4 – (8 – 5) = 4 – 3 = 1 and
(4 – 8) – 5 = – 4 – 5 = -9
Therefore, Subtraction of a – (b – c) ≠ (a – b) – c
(iv) Associative property of Division
In the division of natural numbers, when grouping is changed the result changes. Associative property of division is not true for division.
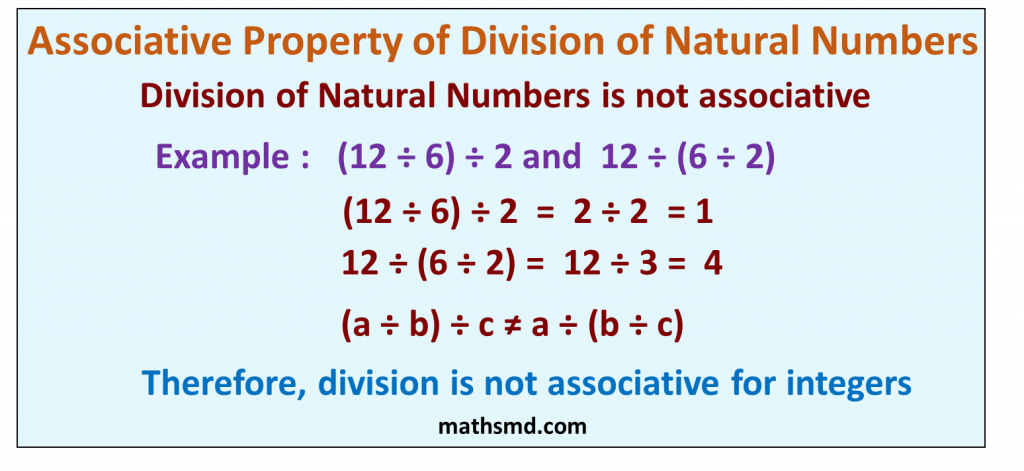
Division: a ÷ (b ÷ c) ≠ (a ÷ b) ÷ c
2 ÷ (3 ÷ 9) = 6 and
(2 ÷ 3) ÷ 9 = 2/27
∴ a ÷ (b ÷ c) ≠ (a ÷ b) ÷ c
This shows that set of natural numbers are not associative under subtraction and division.
This shows that the set of natural numbers N is associative under addition and multiplication but this is not associative under subtraction and division.
(3) Commutative Property
This shows that the sum of two natural numbers remains same even if interchange the order of a numbers.
(i) Commutative Property of Addition

a + b = b + a
Example: 9 + 7 = 16 and
7 + 9 = 16
16 = 16
(ii) Commutative Property of Multiplication
This shows that the product of two natural numbers remains same even if we interchange the order of numbers.
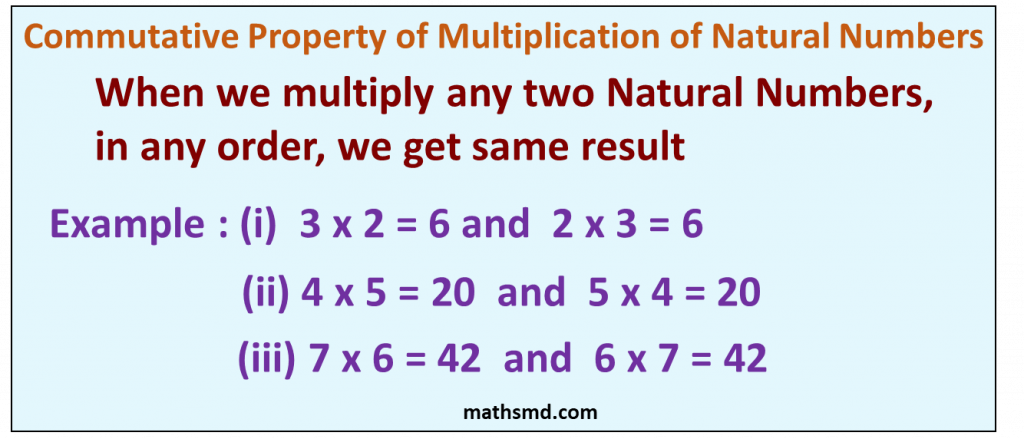
a x b = b x a
Example: 9 x 7 = 63 and
7 x 9 = 63
Addition and multiplication of natural numbers show the commutative property.
(iii) Commutative Property of Subtraction
The set of natural numbers is not commutative under subtraction.
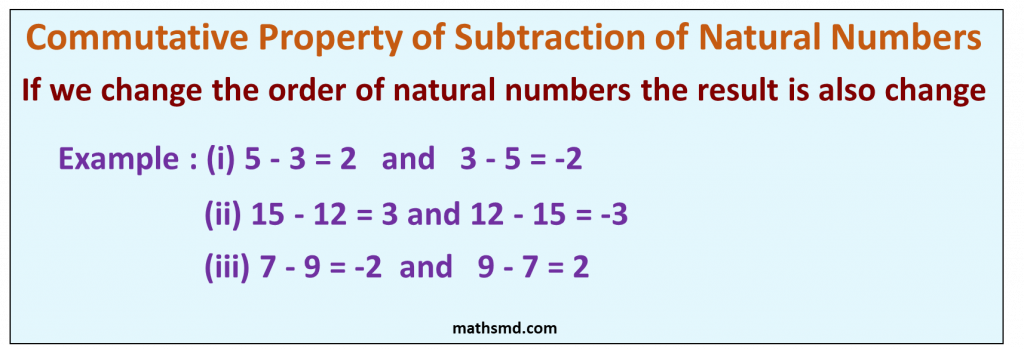
a – b ≠ b – a
Example: 7 – 3 = 4 and
3 – 7 = – 4
4 ≠ -4
∴ a – b ≠ b – a
(iv) Commutative Property of Division
The set of natural numbers is not commutative under division.

a ÷ b ≠ b ÷ a
10 ÷ 5 = 2 and
5 ÷ 10 = 1/2
2 ≠ 1/2
10 ÷ 3 = 3 . 3333….
∴ a ÷ b ≠ b ÷ a
Subtraction and division of natural numbers do not show the commutative property.
This shows that the set of natural numbers N is commutative under addition and multiplication but this is not commutative under subtraction and division.
(4) Distributive Property
Multiplication of natural numbers is always distributive over addition.
(i) Distributivity of multiplication of over Addition
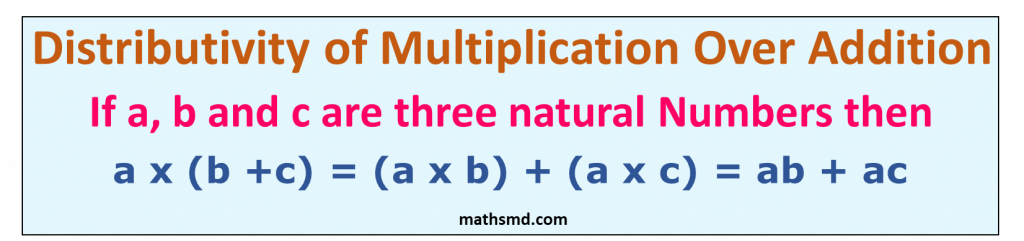
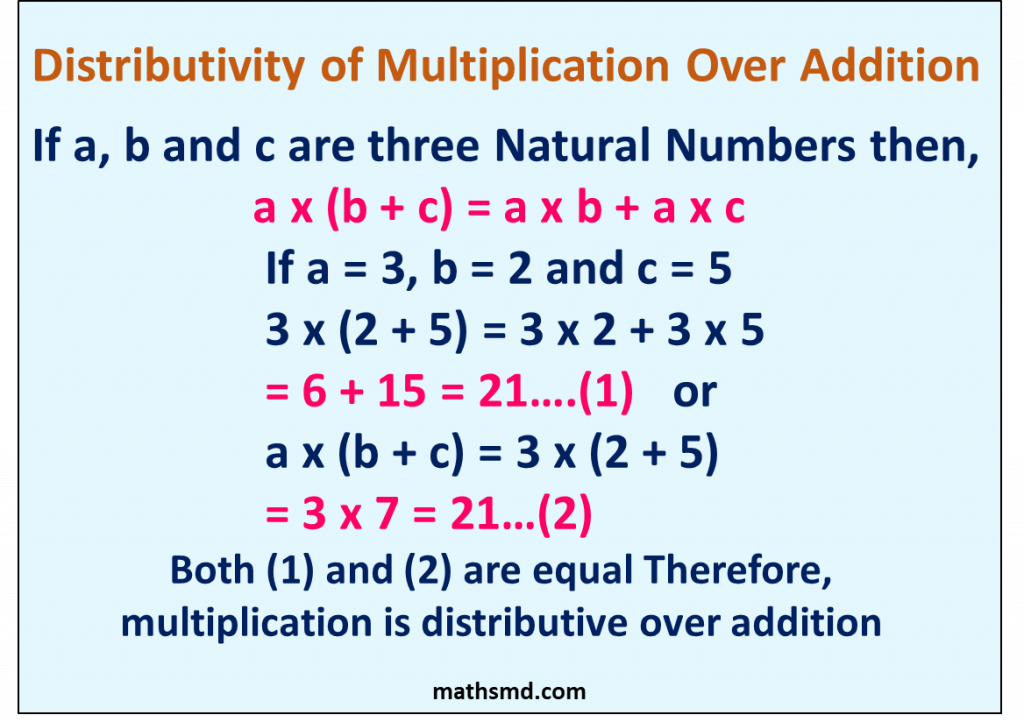
Example : a x (b + c) = ab + ac
Example : 5 x (3 + 4)
= 5 x 3 + 5 x 4
= 12 + 20 = 35
5 x (3 + 4)
= 5 x 7 = 35
(ii) Distributivity of multiplication of over Subtraction
Multiplication of natural numbers is also distributive over subtraction.
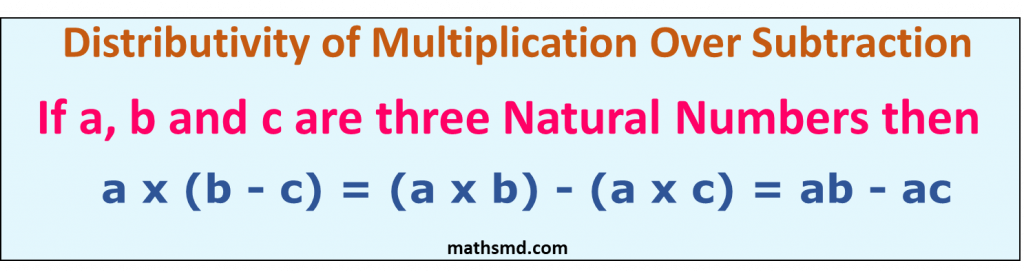

Example : a x (b – c) = ab – ac
Example : 6 x (9 – 4)
= 6 x 9 – 6 x 4
= 54 – 24
= 30
6 x (9 – 4)
= 6 x 5
= 30
