Multiply Fractions with whole numbers

We know that multiplication is repeated addition of the same number. So, multiplying a fraction with a whole number is equal to the whole number times the fraction is added.

Let us understand with an example.

Let us see the steps to multiply a given fraction with a whole number.

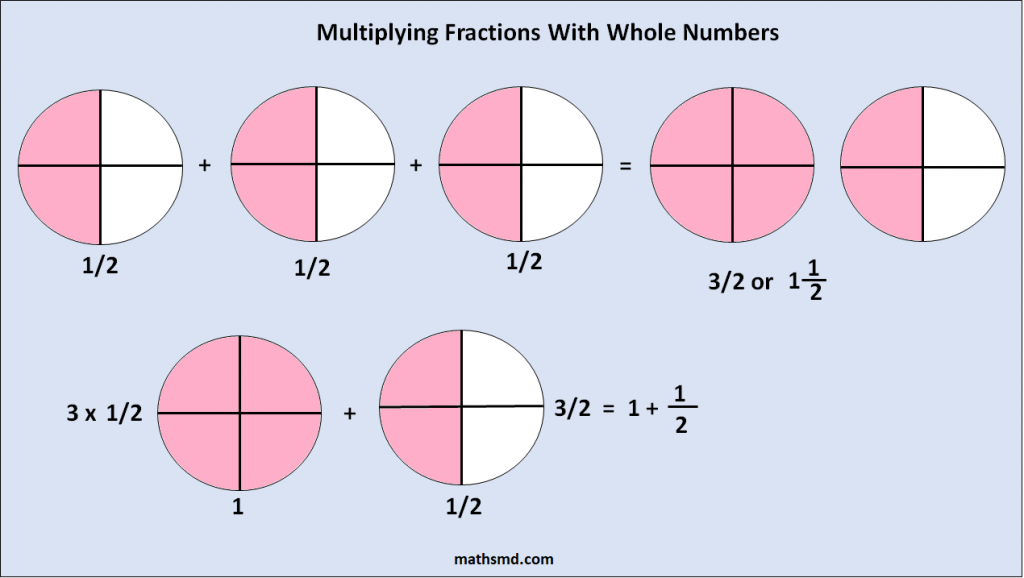
Example: 3 x 1/2

Step 1: Write the whole number in the fractional form by placing ‘1’ in the denominator.

Here 3 is a whole number that can be written as 3/1, now we can multiplied it as a regular fraction.

Now the question is multiply 3/1 x 1/2

Step 2: Multiply the numerators of the fractions.

3 x 1 = 3

Step 3: Multiply the denominators of the fractions.

1 x 2 = 2

The answer is 3/2. This can not be reduced.

means 3 times of 1/2. So, if we add 3 times 1/2 we get result.

3/2 is a improper fraction, so we will change it into a mixed fraction. 7/2 = 3 1/2.

Step 4: Simplify/reduce the answer if needed/possible.

Example: 9 x 1/4

Here 9 is a whole number that can be written as 9/1, now we can multiplied it as a regular fraction.

9/1 x 1/4

= (9 x 1)/(1 x 4)

= 9/4

means 9 times of 1/4.

So, if we add 9 times 1/4 we get result.

9/4 is a improper fraction, so we will change it into a mixed fraction. 9/4 = 2 1/4.

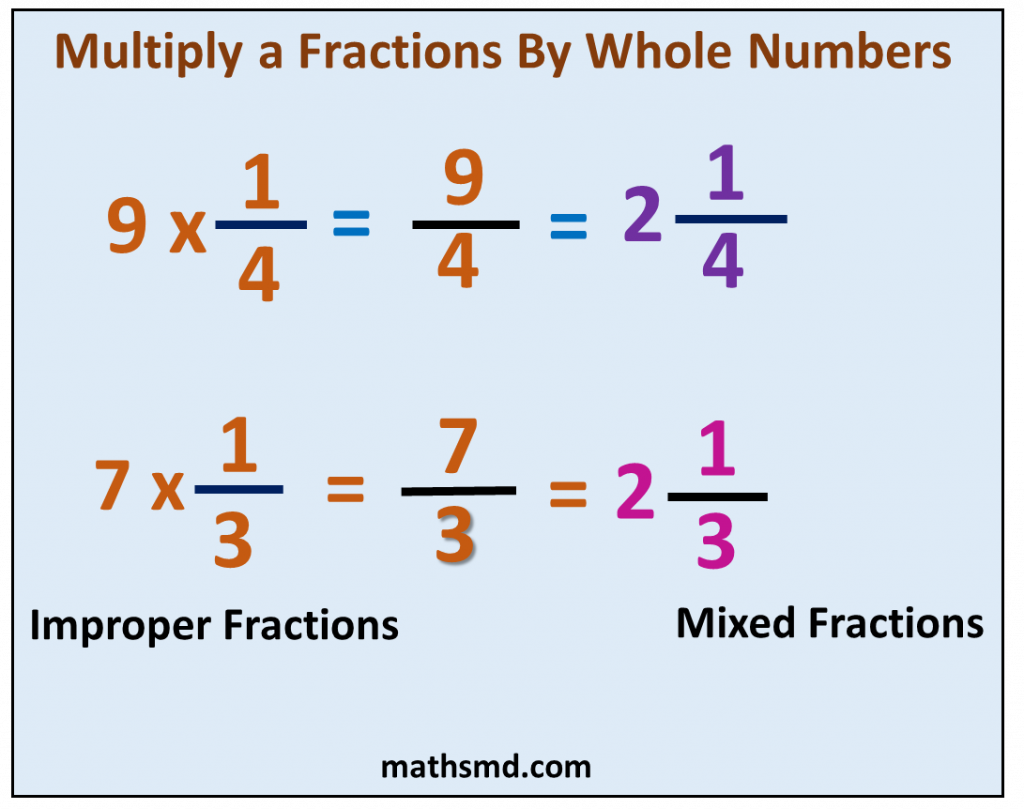
Example: 7 x 1/3

Here 7 is a whole number that can be written as 7/1, now we can multiplied it as a regular fraction.

7/1 x 1/3

= (7 x 1)/(1 x 3)

= 7/3

means 7 times of 1/3.

So, if we add 7 times 1/4 we get result.

7/3 is a improper fraction, so we will change it into a mixed fraction. 7/3 = 2 1/3.