Fractions – Multiplication

Multiplication of fractions: When we multiply any two fractions then the top numbers of the fractions(numerators) and the bottom numbers of the fractions(denominators) are multiplied together.

Example: 1/2 x 3/4

= (1 x 3)/(2 x 4)

= 3/8

How to Multiply Fractions?

Multiplication of fractions is not like as the addition and subtraction of fractions.

When we solve the addition and subtraction of fractions the denominators should be same, but in multiplication, any two fractions with different denominators are easily multiplied.

Let us see an example of multiplication of two fractions in steps.

Step 1: Multiply the numerators.

Step 2: Multiply the denominators.

Step 3: Simplify the resultant fraction to its lowest form.

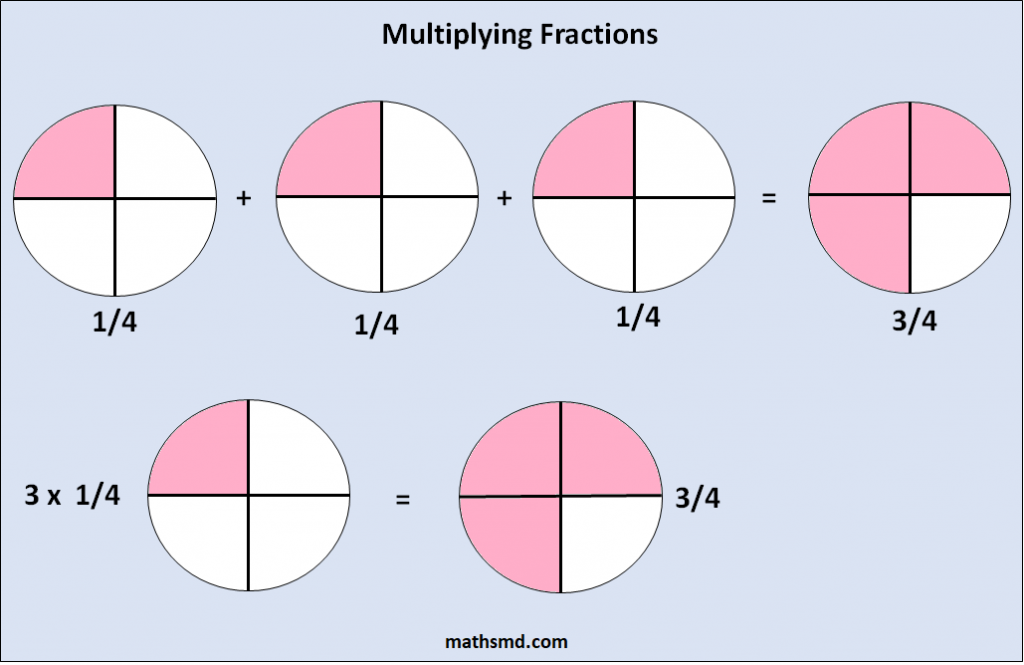
Example: Multiply the fractions 3/1 x 1/4

We start from multiplying the numerators, 3 x 1 = 3

Now multiply denominators, 1 x 4 = 4

= (3 x 1)/(1 x 4)= 3/4

Example: Multiply the fractions 1/5 x 5/3

We start from multiplying the numerators, 1 x 5 = 5

Now multiply denominators, 5 x 3 = 15

= (1 x 5)/(5 x 3)

= 5/15

Now simplify this value in its lowest form.

The greatest common factor of 3 and 15 is 3, so to simplify the fraction 5/15 divide both 3 and 15 by 3.

Therefore, 5/15 = 1/3

∴ 1/5 x 5/3 = 1/3

If a/b and c/d are two fractions, then the multiplication of a/b and c/d will be

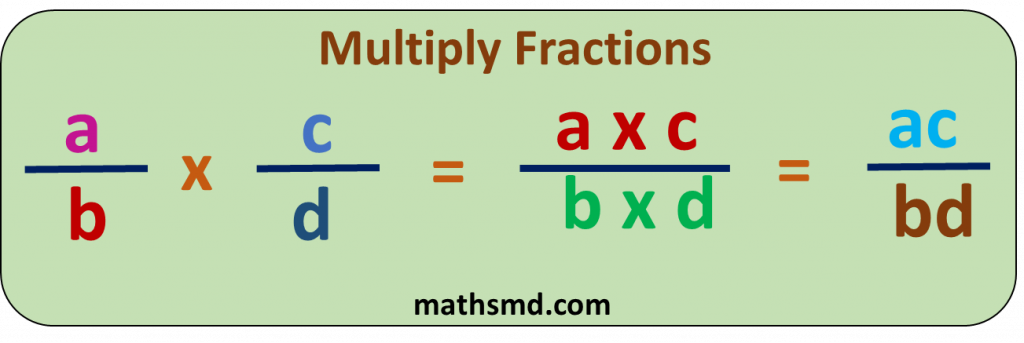
(a/b) x (c/d) = (a x c)/(b x d)

= (ac)/(bd)

Where b and are not equal to zero.