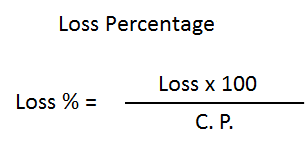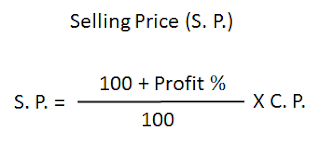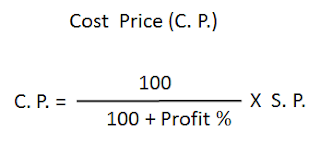Problems – Solutions – Profit and Loss – Formulas

Important Facts

The cost price is the price at which a product orservice is purchased by seller.

The selling price is the price at which a product or service is sold to the buyer.

When the selling price (SP) is greater than cost price(CP) the profit or gain.

Selling price (SP) > Cost price = Profit or Gain

Profit = Selling price (SP) – Cost price (CP)

Profit or Gain – When the difference between selling price (SP) and cost price (CP) is positive, that amount is called profit or gain.

If selling price of the product or service is more than its cost price, then seller makes a profit.

To find the profit percent the formula is

When the selling price (SP) is less than cost price (CP) 

Selling price (SP) < Cost price = loss

Loss = Cost price (CP) – Selling price (CP)

Loss – The difference between cost price (CP) and selling price (SP) is positive, that amount is called Loss.

If selling price of the product or service is less than its cost price, then seller suffers a loss in it.

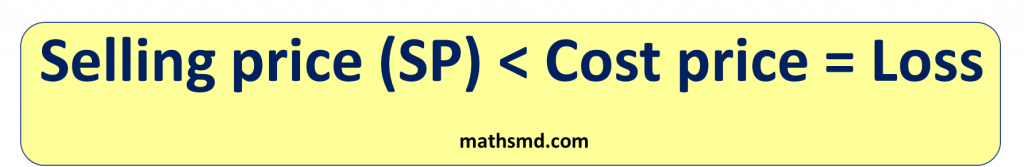
To find the loss percent the formula is

Formula’s

1. John bought a book for $ 400 and sold it for $ 500. Find John’s profit percent.

Reading this question we observe that CP of book is $ 400 and SP of the book is $500, from this we come to the conclusion that the john has made a profit on purchasing as SP > CP. So the formula will be

Solution: Here, cost price (CP) of the book = $ 400

and sell price (SP) of the book is = $ 500

Since (SP) > (CP), John makes a profit

     Profit = Selling price (SP) – Cost price (CP)

                    Profit = $ (500 – 400)

 = $ 100

              Profit % = {(100/400) x 100} %

                              = {(100 x 100)/400} %

                              = (100/4) %

                              = 25 %

Answer is John’s profit percent = 25 %

2. Mike bought a DVD $ 850 and sold it $ 900. Find his profit or loss percent. 

Solution: Here, cost price (CP) of the DVD = $ 850

and sell price (SP) of the DVD is = $ 900

Since (SP) > (CP), Mike makes a profit

Profit = Selling price (SP) – Cost price (CP)

                    Profit = $ (900 – 850)

= $ 50

                 Profit % = {(50/850) x 100} %

                              = {(50 x 100)/850} %

                              = (100/17) %

                              = 5.88 %

Answer is Mike’s profit percent = 5.88 %

3. Jack bought a pen $ 25 and sold it $ 90. Find his profit or loss percent. 

Solution: Here, cost price (CP) of the pen = $ 25

and sell price (SP) of the pen is = $ 90

Since (SP) > (CP), Mike makes a profit

Profit = Selling price (SP) – Cost price (CP)

                    Profit = $ (90 – 25)

= $ 65  

                 Profit % = {(65/25) x 100} %

= {(65 x 100)/25} %

                              = (65 x 4) %

                              = 260 %

Answer is Jack’s profit percent = 260 %

4. Robert bought a TV $ 5600 and sold it $ 9700. Find his profit or loss percent.

Solution: Here, cost price (CP) of the TV = $ 5600

and sell price (SP) of the TV is = $ 9700

Since (SP) > (CP), Mike makes a profit

Profit = Selling price (SP) – Cost price (CP)

                    Profit = $ (9700 – 5600)

= $ 4100

              Profit % = {(4100/5600) x 100} %

= {(4100)/56} %

                              = (73.21) %

                              = 73.21 %

Answer is Robert’s profit percent = 73.21 %

5. Ron bought a pencil $ 50 and sold it $ 40. Find his profit or loss percent. 

Solution: Here, cost price (CP) of the pencil = $ 50

and sell price (SP) of the pencil is = $ 40

Selling price (SP) < Cost price = loss

Since (SP) < (CP), Ron makes a loss

Loss = Cost price (CP) – Selling price (CP)

loss = $ (50 – 40)

= $ 10

                 Loss % = {(10/50) x 100} %

= {(1000)/50} %

                              = (20) %

                              = 20 %

Answer is Ron’s loss percent = 20 %

6. A Bike was sold for $ 15000 at a loss of 25%. What is the Cost price of the bike?

Solution: We can easily solve this problem by given list of formula’s, put the value in appropriate formula from the list of profit and loss formula’s.

The formula is 

put the value in the formula, we get

               CP = {100/(100 – 25)} x 15000

                  CP = (100/75) x 15000

                  CP = (100 x 15000)/75

                 CP = (4 x 15000)/3

                 CP = 60000/3

                  CP = 20000

Therefore, Cost price of the bike is $ 20000.

When we solve the profit percent and loss percent problems, sometimes after purchasing a product, we have to pay some more money for things like repairing charges, transportation, local taxes etc. These extra expenses are called overheads.

Therefore, calculating the total cost price, we add overheads to the purchase price.

7. Ron purchased an old bike for $ 15000 and spent $ 2500 on its overhauling, then sold it to Maddy for $ 16000. Find his profit or loss percent. 

Solution: Cost price of the bike = $ 15000

Overheads = $ 2500

Total cost price = $ 15000 + $ 2500

Total cost price = $ 17500

             $17500 is selling price

Selling price = $ 17500

Since (SP) < (CP), Ron makes a loss.

Loss = Cost price (CP) – Selling price (CP)

Loss = $ 17500 – $ 16000

Loss = $ 1500

              Loss% = {(1500/17500) x 100}%

                          = $ 8.57 %

Therefore, loss percent of the bike is $ 8.57 %.

8. Robert bought an almirah for $ 8250 and spent it $ 475 on its repairs. Then he sold it for $ 9500. 
Find his profit or loss percent.

      Solution: Cost price (CP) of the almirah = $ 8250

                       Repair charge = $ 475

          Total cost price = $ 8250 + $ 475

                                 = $ 8725

               Selling price = $ 9500

          (SP) > (CP), Robert makes profit.

         Profit = Selling price (SP) – Cost price (CP)

Profit  = $ 9500 –  $ 8725

Profit  = $ 325

                 Profit % = (325/8725) x 100

                  Profit % = $3.72 %