Angle Bisector – Definition -Examples 

The angle(interior) bisector of an angle is a line or line segment that divides the angle into two (congruent)equal parts. 

The angle bisector is also called the internal angle 
bisector.

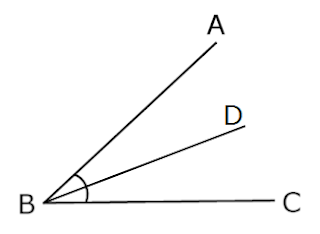
In ∠ABC, BD is angle bisector of ∠ABC.

In above figure BD is bisect the ∠ABC.

∵ ∠ABC = 90⁰
∴ ∠ABD   = 45⁰
and ∠DBC = 45⁰

∠ABD and ∠DBC are congruent

∴ ∠ABD = ∠DBC

Note- Any point on angle bisector is equidistant from the to sides of the angle.

In below figure BD is angle bisector of ∠ABC. Find the measure of ∠ABC.

BD is an angle bisector, of ∠ABC, 

∴ ∠CBD = ∠ABD

∵  ∠CBD = 35⁰

∴ ∠ABD = 35⁰

Therefore, ∠CBD = 35^0,  BD is angle bisector

∵ ∠ABC = ∠ABD + ∠CBD

Substituted the value of ∠ABD and ∠CBD

∴ ∠ABC = 35⁰ + 35⁰
  ∴  ∠ABC = 70⁰