Irrational Numbers – Definition & Examples
Irrational Numbers – Definition, Examples, Properties
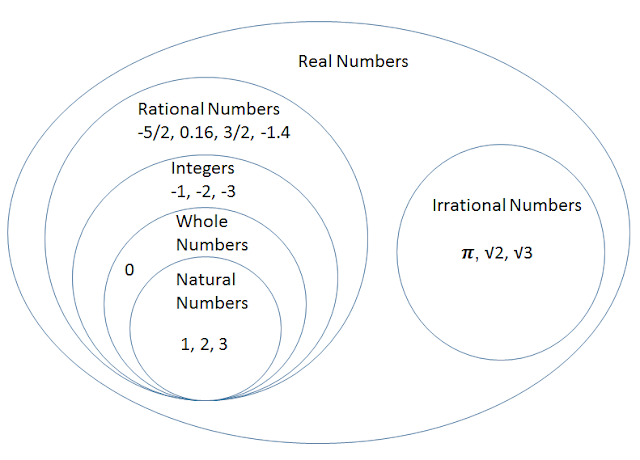
Definition and Properties of Irrational Numbers
Irrational Numbers
An irrational number is a real number that cannot be expressed as a simple fraction, i.e., it cannot be written in the form a/b, where a and b are integers and b≠0.
This means irrational numbers have non terminating, non-repeating decimal expansions.
An “Irrational Number” is a Real Number that cannot be expressed as a simple fraction for any Integers. Simply Irrational means not Rational.
2.5 = 5/2 is a ratio or fraction, but
𝝅 = 3.1415926…is not a ratio or fraction.
Historical Background
The concept of irrational numbers was first discovered by the ancient Greeks. Hippasus, a disciple of Pythagoras, is often credited with the discovery when he demonstrated that ✓2 could not be expressed as a fraction, thus revealing the existence of numbers that are not rational.
Examples of Irrational Numbers
(1) Square Roots of Non-Perfect Squares:
- ✓2 (approximately 1.4142135…)
- ✓3(approximately 1.7320508…)
(2) Transcendental Numbers:
- π (approximately 3.14159265…)
- e (approximately 2.7182818…)
(3) Other Examples:
- The golden ratio
- ϕ (approximately 1.6180339…)
- π (Pi): The ratio of the circumference of a circle to its diameter.
- √2 (Square Root of 2): The length of the diagonal of a square with side length 1.
- e (Euler’s Number): A mathematical constant equal to approximately 2.71828.
Proof of Irrationality: To prove that a number is irrational, one typically uses a proof by contradiction.
For example, to prove √2 is irrational, assume the opposite (that √2 is rational), and then derive a contradiction showing that this assumption cannot be true.
Properties of Irrational Numbers
Non-Terminating and Non-Repeating Decimals:
Non-terminating: The decimal representation of an irrational number goes on forever without repeating any pattern.
Non-repeating: Unlike rational numbers, which have repeating decimal patterns or terminate (end), irrational numbers have decimal expansions that do not settle into a repeating sequence of digits.
Closure under Certain Operations:
Irrational numbers are closed under addition and multiplication only in specific cases.
For example, the sum of two irrational numbers can be rational (e.g., ✓2+(−✓2)=0) or irrational. Similarly, their product can be rational (e.g., ✓2⋅✓2=2) or irrational.
Examples:
Unlike rational numbers, which either terminate (e.g., 1/2 = 0.5= or repeat (e.g., 0.3333…= 1/3), the decimal expansion of irrational numbers never ends and never forms a repeating pattern.
Dense in the Real Numbers:
Between any two real numbers, no matter how close, there is always at least one irrational number. This property shows that irrational numbers are densely distributed along the number line.
Proving Irrationality
Proof by Contradiction
Example: Proving ✓2 is Irrational:
Assume ✓2 is rational. Then, it can be expressed as
a/b where a and b are integers with no common factors other than 1, and b≠0.
✓2 = a/b
Squaring both sides:
2 =a2/b2 ⟹a2=2b2
This implies a2 is even, and hence a must be even (since the square of an odd number is odd). Let
a=2k for some integer k.
Substituting back:
(2k)2=2b2⟹4k2=2b2⟹b2=2k2
Thus, b2 is even, and b must also be even. But if both
a and b are even, they share a common factor of 2, contradicting our initial assumption that they have no common factors other than 1. Therefore,
✓2 cannot be rational and must be irrational.
Decimal expansions of irrational Numbers does not repeat or terminate.
Example: √2 and √2 are famous Irrational Numbers.
𝝅 = 3.14159265358979323846264338327950…(etc).
We cannot write 𝝅 into a simple fraction form, approximate value of 𝝅 is 22/7.
22/7 = 3.14159265358979323846264338327950…
is closed but not accurate value of 𝝅.
𝝅 cannot be written in a fractional or rational form so, it is Irrational.
√2 = 1.4142135623730950488016887242096…(etc).
√2 is also cannot be written in a fractional or rational form so, it is Irrational.
Some more examples;
Number Fraction Rational or Irrational
1.25 5/4 Rational
.003 1/3000 Rational
𝝅 22/7(approximate) Irrational
√2 ? Irrational
Significance in Mathematics
Irrational numbers play a crucial role in various fields of mathematics, including number theory, algebra, and calculus.
They ensure the completeness of the real numbers, meaning that every non-empty set of real numbers that is bounded above has a least upper bound in the real numbers.
This property is essential for the rigorous formulation of limits, continuity, and differentiability in calculus.
They challenge our understanding of numbers and are essential in various theoretical and practical applications, including computer science, physics, and engineering.
Conclusion: Understanding irrational numbers enriches our comprehension of the infinite and non-repeating nature of numbers. They are a cornerstone in mathematics, highlighting the diversity and complexity within the realm of real numbers
Irrational numbers are a fundamental component of the real number system, distinguished by their non-terminating, non-repeating decimal expansions and their inability to be represented as fractions. They are densely distributed among real numbers and are vital for the structure and completeness of the real number line.