Property of Integers – Divisive Identity

What is Divisive Identity Property

The divisive identity property is one of the important property of division of numbers.

This property states that if we divide any, number by 1 the result will be the number itself.

Dividing any number by 1 equals the number itself.

Divisive Identity Property

The divisive identity for integers states that if a number is divided by 1 the division will be the number itself.

Therefore, 1 is called the divisive identity for integers.

For any Integer a, a ÷ 1 = a

Therefore, one is the multiplicative identity for integers.

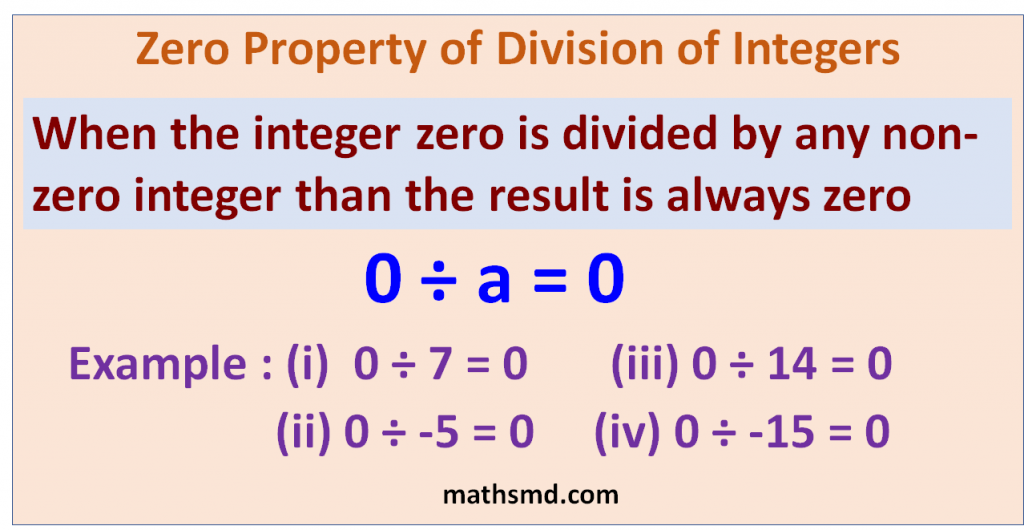
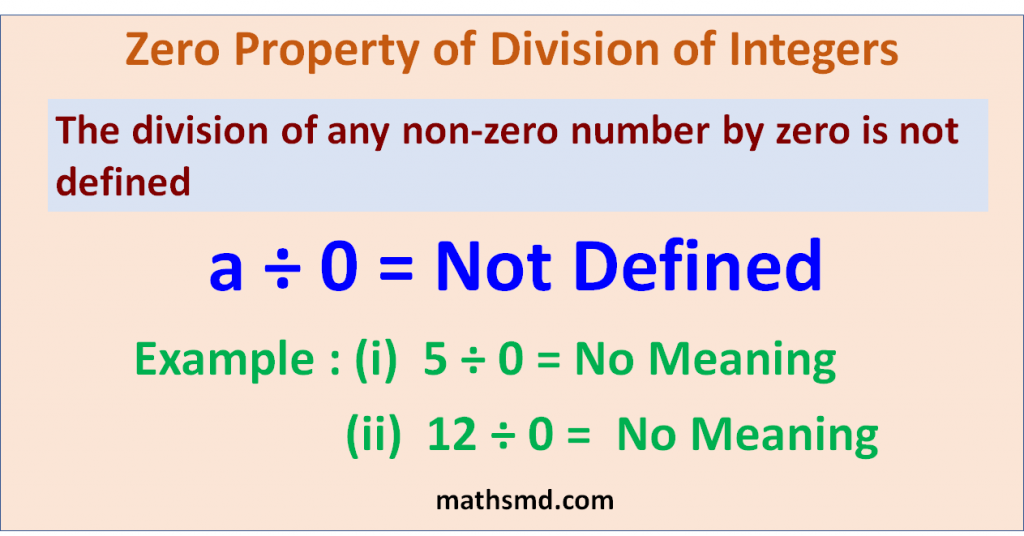
Division of any non-zero number by zero is meaningless, because division by zero is not defined.

Example: 3 x 0 = 0, -5 x 0 = 0,therefore, any non zero integer a x 0 = 0 x a = 0

Zero division property

Division of any non-zero number by zero is meaningless. Division by zero is not defined.

Identity Property: Identity Property states that when any zero is added to any integer it will give the same given number. Zero called additive identity. For any integer a, a + 0 = 0

Therefore, 1 is also called divisive identity.

This property is also true for multiplication, because multiplying 1 to any number equals the number itself.