Property of Integers – Commutative

Addition

The commutative property of addition states that, two integers can be added in any order, the result will be the same. Order of terms doesn’t matter, swapping of terms will not change the sum.

Example: 9 + (-5) = 4 and

(-5) + 9 = 4

So, 9 + (-5) = (-5) + 9 = 4

both are equal.

Therefore, For any two integers ‘a and b’ we can say

a + b = b + a

addition of integers is commutative.

Subtraction

Subtraction is not commutative for integers. In subtraction expression, if we change the order of integers the result is also change.

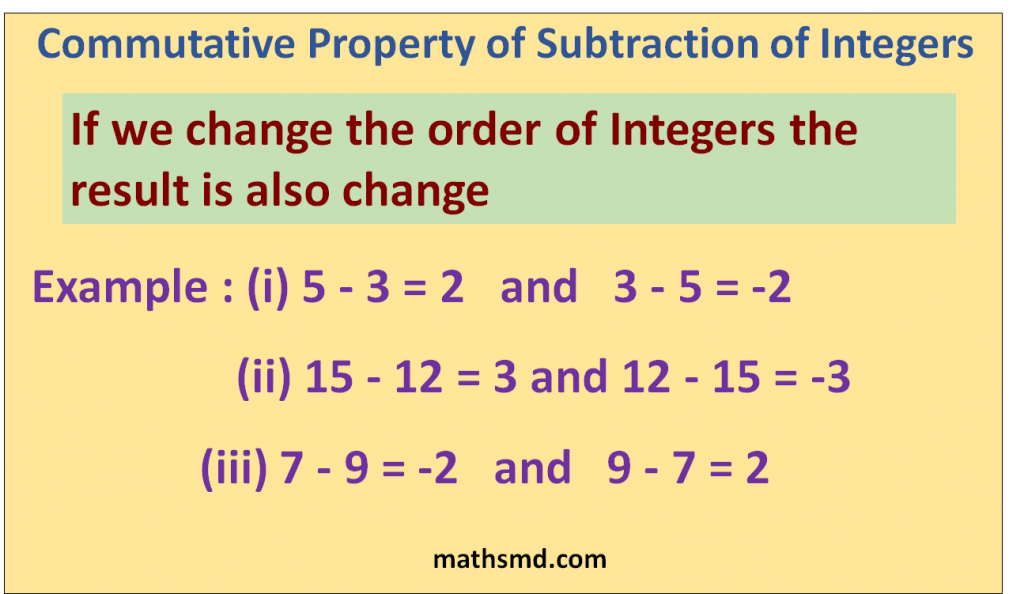
Commutative Property for subtraction of Integers understand with the help of examples.

Example: Explain commutative property for subtraction of integers

(i) 4 and -7,

4 – (-7) = 4 + 7 = 11 and

(-7) – (-4) = -7 + 4 = -3

11 ≠ -3

Both result are different, so we can say that subtraction is not commutative for integers.

(ii) -5 and 12,

-5 – 12 = -5 – 12 = -17 and

(-5) – (-12) = -5 + 12 = 9

-17 ≠ 9

Both result are different, so we can say that

subtraction is not commutative for integers.

Multiplication

Commutative Property of multiplication states that, if we multiply two integers, in any order we get same result. It doesn’t matter which order the numbers are multiplied, any case we will get same answer.

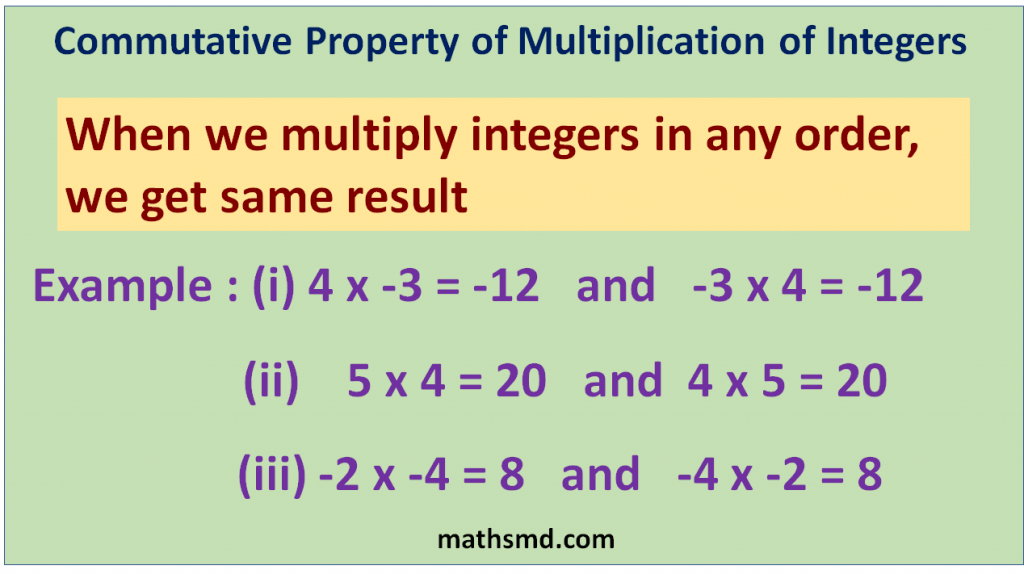
Example: (i) 4 x 3 = 12  and

  3 x 4 = 12 

Here, we multiplying three and four together we get 12, and if we multiplying four and three together we get 12.

(ii) 5 x 4 = 20 and

4 x 5 = 20

(iii) (-3) x (-2) = 6 and

(-2) x (-3) = 6

It is true for 8 and 9 and 7 and 6 also. So, we can multiply integers in any order.

Therefore, multiplication is commutative for  integers. The property is known as commutative property of multiplication.

Therefore, the

set of integers is closed under multiplication.

If a and b are two integers then

a x b = b x a

Division

Commutative Property under division states that division of two integers does not always result in an integer.

Division is not commutative for integers.

Observe the following examples:

12 ÷ 3 = 4 and 3 ÷ 12 = 1/4

Therefore, 12 ÷ 3 ≠ 3 ÷ 12

From above examples, we can say that

integers are not commutative under division.