Closure Property of Natural Numbers
Closure Property of Natural Numbers
The closure property is a fundamental concept in mathematics that applies to various operations within a set. When we say that a set is closed under an operation, we mean that performing that operation on elements within the set always results in an element that is also within the set. For natural numbers, the closure property can be explored with addition and multiplication.
Natural Numbers
Natural numbers are the set of positive integers starting from 1, 2, 3, and so on. In mathematical notation, they are represented as: N={1,2,3,4,5,…}
Closure Property with Addition
Definition: The set of natural numbers is closed under addition if, for any two natural numbers a and b, the sum a+b is also a natural number.
Example:
- Let a=3 and b=5.
- 3+5=8
- Since 8 is a natural number, the set of natural numbers is closed under addition.
Closure Property with Multiplication
Definition: The set of natural numbers is closed under multiplication if, for any two natural numbers a and b, the product a×b is also a natural number.
Example:
- Let a=4 and b=6.
- 4×6=24
- Since 24 is a natural number, the set of natural numbers is closed under multiplication.
Visual Examples
To illustrate the closure property, let’s look some examples:
Closure Property with Addition
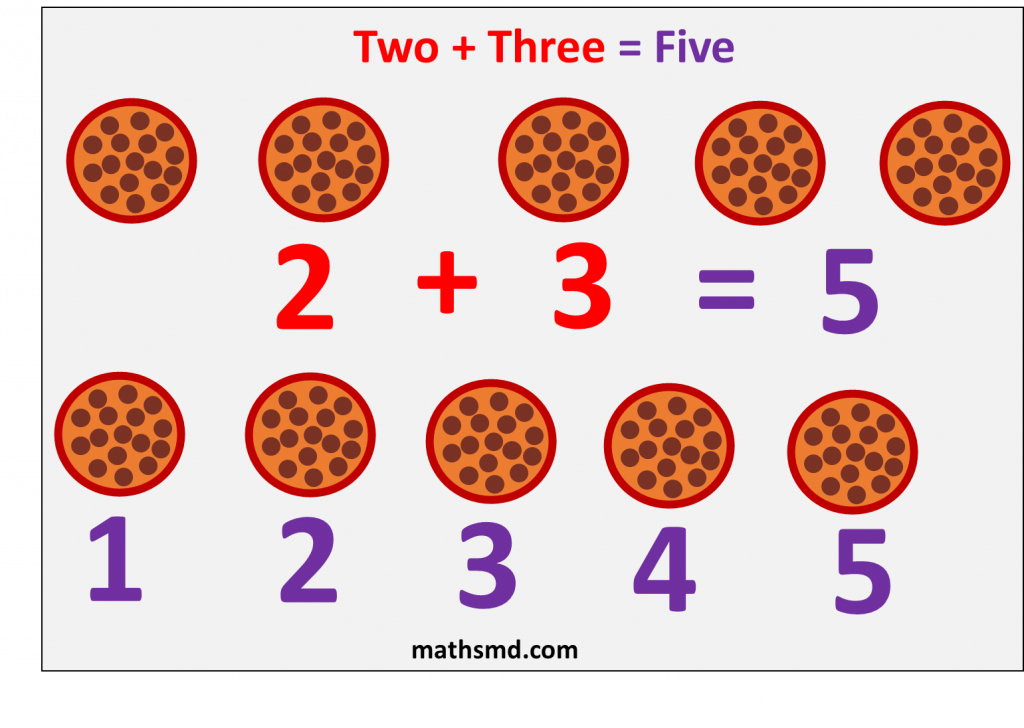
- Example with Numbers:
- The addition of two natural numbers 3 and 2, resulting in 5, which is also a natural number.
- Visual Addition:
- This visual shows how adding 3 cookies to 2 cookies results in 5 cookies, emphasizing that the result is still a countable number of cookies, hence a natural number.
Closure Property with Multiplication
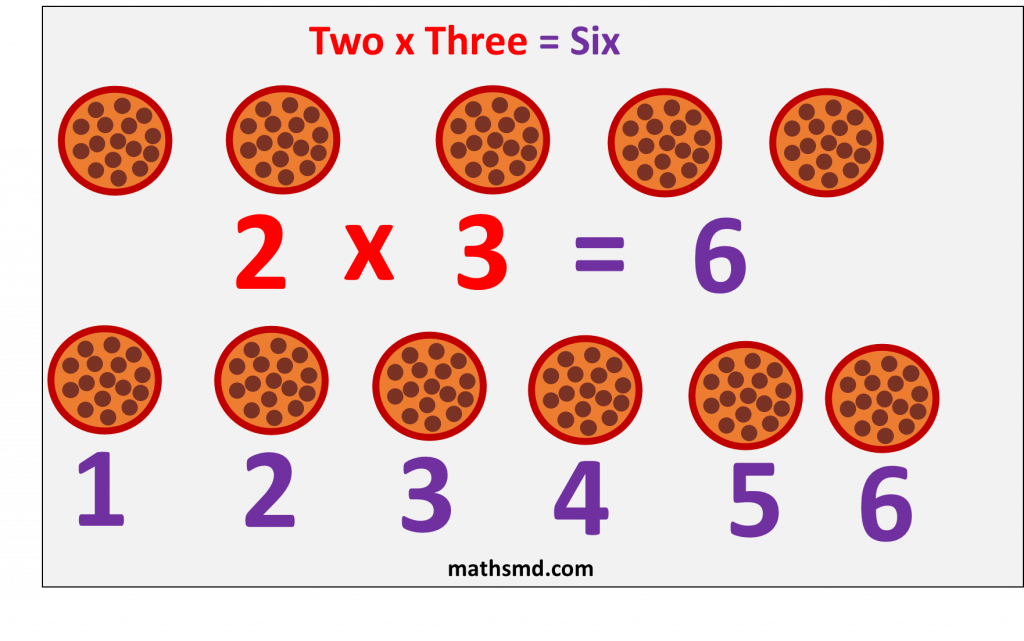
- Example with Numbers:
- Here, multiplying 2 by 3 results in 6, which is also a natural number.
- Visual Multiplication:
- This image depicts multiplying groups of objects, showing that the product is still a natural number.
Summary
The closure property is an essential aspect of natural numbers, ensuring that the operations of addition and multiplication within the set of natural numbers result in outcomes that are also within the same set. This property provides a foundation for more complex mathematical concepts and operations.