Equivalent Rational Numbers – Definition – Examples

Rational Numbers

A rational number can be represented in the form of p/q, where q is not equal to zero.

Equivalent Rational Numbers

Two rational numbers are said to be equivalent, if they have same value but can be represented in different forms.

We know that if we multiply or divide the numerator and denominator of a fraction by same positive integer, the value of the fraction does not change and we get equivalent fractions of the given fractions.

Let a/b is a rational number, then (a x m)/(b x m) is a rational number which is equivalent to a/b, where, m is a non-zero integer.

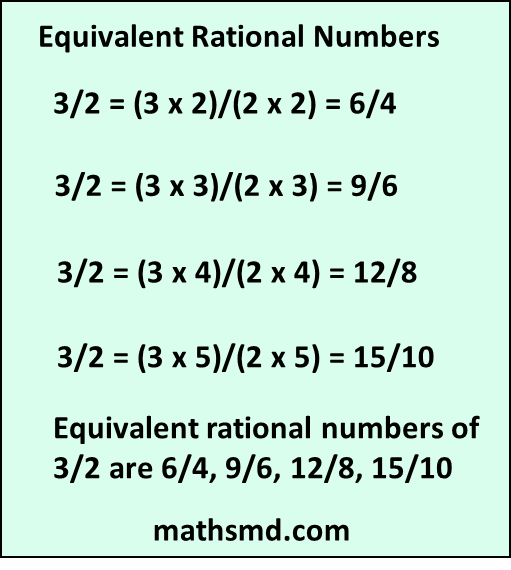
Example: Find equivalent rational numbers of 1/2.

Equivalent rational numbers of 1/2 are 2/4, 3/6, 4/8 and so on.

Let a/b is a rational number, consider common divisor m, then (a ÷ m)/(b ÷ m) is a rational number which is equivalent to a/b.

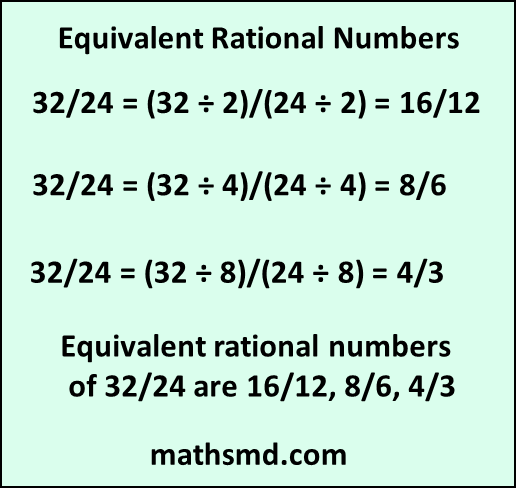
Example: Find equivalent rational numbers of 24/36.

Equivalent rational numbers of 24/36 are 12/18, 6/9, 2/3 and so on.