Division of Two Rational Numbers

In this tutorial, we will learn, division of rational numbers.

Rational numbers are written as fractions, therefore to divide a given rational number by another rational number, we have to multiply the given rational number by the reciprocal of the second rational number.

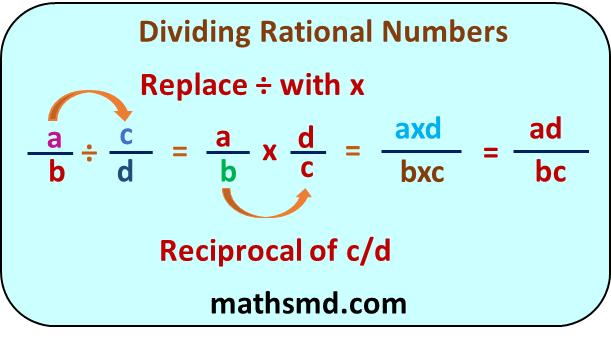
Let a/b is a rational number then its reciprocal will be b/a.

Any two rational numbers can be divided by the following method.

Step 1: First, express the given rational number in the form of a fraction.

Step 2: Keep the numerator as it is and multiply with the reciprocal of the denominator in rational number.

Step 3: Find the product of the numerator and the denominator to get the result.

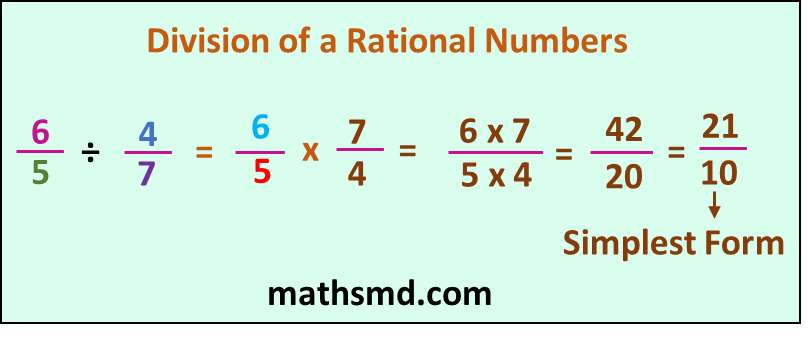
Example:

(1) 2/3 ÷ 5/4 becomes 2/3 x 4/5

= 2/3 x 4/5

= (2 x 4)/(3 x 5)

= 8/20

= 2/5

That is, division is simply divisor value can be reciprocated and multiplied by the numerator.

Remember

• Zero divided by any rational number result is always equal to zero.

• The product of a rational number with its reciprocal is always 1.

• The reciprocal of a rational number is called the multiplicative inverse of rational number.

• Zero has no reciprocal as,

reciprocal of 0 = 0/1 = 1/0 which is not defined.

• When a rational number (except zero) is divided by another rational number (except zero) the quotient is always a rational number.

• Division of any rational number by itself gives the quotient 1.

• When a rational number is divided by 1, the quotient is a rational number itself.

• Dividing a positive rational number and a negative rational number will result in a negative quotient.

• Dividing two positive rational numbers will result in a positive quotient.

• Dividing two negative rational numbers will result in a positive quotient.

• Reciprocal of a positive rational number is positive and reciprocal of a negative rational number is negative.