Complex Numbers – Definition and Examples

Complex Numbers

The concept Complex numbers was first used in 1st century by a Greek mathematician Alexandria, when he tried to find the square root of a negative number. After that in 16th century complex number was define by Italian mathematician Gerolamo Cardano, in the process of finding the negative roots of cubic and quadratic polynomial expressions.
Complex numbers are helpful in finding the square root of negative numbers.

A complex number is the addition of a real number and an imaginary number i. e., one part is purely real and other part is purely imaginary.

What are real numbers?

In number system any number which is present such as positive, negative, zero, rational, irrational, fractions, etc. are real numbers.

Example: 13, -38, 0, 5/4, 9.7, etc., all are real numbers.

What are Imaginary numbers?

The numbers which are nor real are called imaginary numbers.

Example: √-7, √-10, √-11, √-27 all are imaginary numbers.

What are Complex Numbers?

Complex number is combination of a real number and an imaginary number.

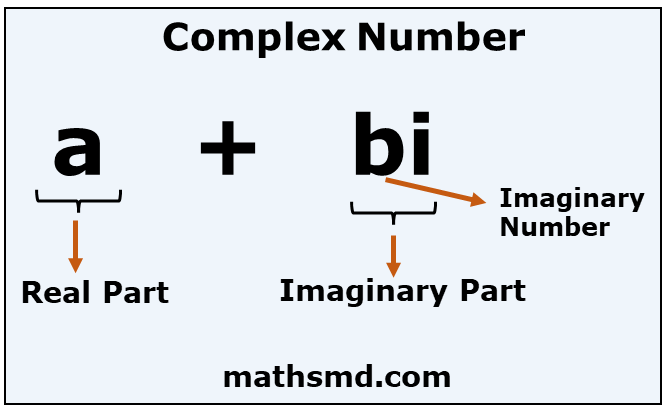
z = a + bi is a complex number, then a and b are called real and imaginary parts respectively, of the complex number and usually represented by z. written as Re(z) =a and Im(z) = b.

Two complex numbers z1 = a +ib and z2 = c +id are said to be equal, if a =c and b = d.

In number system, complex number is an element that contains the real numbers and a specific element denoted i, called an imaginary number whose square is equal to √-1, and satisfying the equation i² = -1.

Every complex number can be expressed in the form a + bi, where a and b are real numbers. Because no real number satisfies the above equation.

The square of i is -1.

Set of Complex Numbers

The set of complex numbers is denoted by C.

Complex numbers are consists two parts a real number and The value of i = √-1 which denotes Iota(Imaginary number).

An imaginary number is usually represented by ‘i’ or ‘j’, which is equal to √-1.

Examples of complex numbers are 1 +j, -15 – 4j, 0.43 + 3.2 i, √5 + √2i, etc,.

The value of a is called the real part which is denoted by Re(z) and b is called the imaginary part Im(z). Also ib number is called an imaginary number.

Therefore, the square of the imaginary number is a negative number.

If we solve square root of 25, we get 5, but if we solve square root of -25 we will not solve it.

There is no real number whose square is -25, but there exists a complex number whose square can be negative.

We define a quantity i = √-1 so its square is -1. so, √-25 = come under 5i.

Example: z = 2 + 3i, Re(z) = 2, Im(z) = 3 and if z = 2 = 2 + 0i is also a complex number as it can be written in the form of a + bi.

This means all real numbers come under complex numbers.

Power of i

i = √-1

i² = -1

i³ = i x i² = i(-1) = -i

i⁴ = (i²)² = (-1)² = 1

Is 0 a complex Number?

We know that 0 is a real number and real numbers are part of complex numbers.Therefore, 0 is a complex number and can be represented as 0 + 0i.