If a transversal intersects two lines, such that a pair of alternate interior angles are equal then the two lines are parallel.

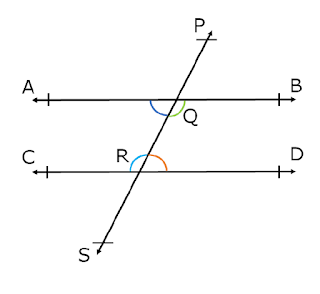
Given: Two lines AB and CD, and PS be transversal intersecting, AB at Q and CD at R.

Each pair of alternate interior angles are equal.

i.e. ∠BQR = ∠CRQ and ∠AQR = ∠QRD

To Prove: AB ∥ CD

Proof: Transversal PS intersecting, lines AB at Q and CD at R.

For lines AB and PS 

∠AQP = ∠BQR  (Vertically opposite angles)….1

but, ∠BQR = ∠CRQ (Given)….2

From (1) and (2)

∠AQP = ∠CRQ

These are corresponding angles,

Therefore, lines AB and CD, with transversal PS, pair of corresponding angles are equal.

so,   AB ∥ CD

Hence proved.