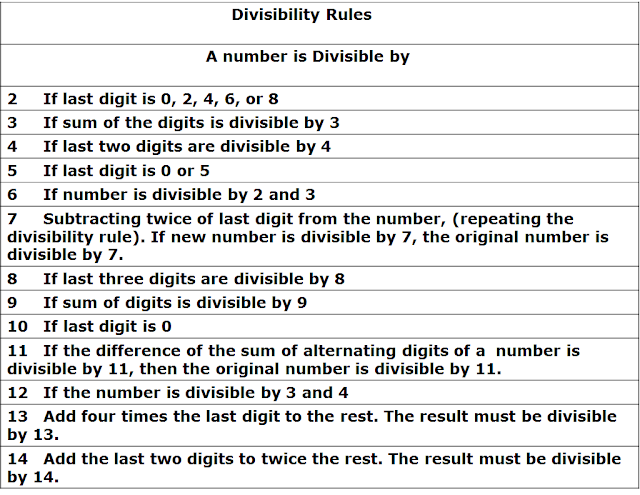
Divisibility Rules For 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13 and 14

Divisibility Rules – Number Divisible by 1

When we divide number 1, to another number, then we get result is a Whole number.

In other words we can say that any number divided by 1 we get the number itself.

Every number is divisible by 1.

Example: 15 is divisible by 1, and the result is 15.

              244 is divisible by 1, and the result is 244.

1, 4, 25, 349, 2689, 34879, 237985, divided by 1, and result is same number in all of numbers.

              Any number /1 = 1

but 32 is not divisible by 5, because 32/5 = 6.4 
6.4 is not a whole number.

Divisibility Rules – Number Divisible by 2

If a number has any of the digit 0, 2, 4, 6, or 8 in its last digit(ones place) is divisible by 2.

In other words,

A number is divisible by 2, if its last digit is 0, 2, 4, 6, or 8.

Example:

(1) 20 is divisible by 2, because its last digit is 0. 

(2) 504 is divisible by 2, because its last digit is 4. 

(3) 726 is divisible by 2, because its last digit is 6

(4) 3648 is divisible by 2, because its last digit is 8.

but 15 is not divisible by 2, because its last digit is not 0, 2, 4, 6, or 8. 

Divisibility Rules – Number Divisible by 3

A number is divisible by 3, If the sum of the digits of the number is divisible by 3.

In other words we can say that sum of the digits of a number is multiple of 3, then the number is divisible by 3.

Example 1: First we take any number suppose the number is 924, 

now add together each digit of the number (9 + 2 + 4). 

we see that the number 924 has total of digits is 15 and 15 is divisible by 3.

15/3 = 5, so 15 is divisible by 3.

Therefore, 924 is divisible by 3

Example 2: Is 27 divisible by 3.

27 has sum of digits 9, which is divisible by 3, so 27 is divisible by 3.

                          2 + 7 = 9, 

                             9/3 = 3

Therefore, 27 is divisible by 3

Example 3: Is 39 divisible by 3,

Solution: sum of digits 39, is 3 + 9 = 12, 

12/3 = 4, 12 is divisible by 3.

 Therefore, 39 is divisible by 3

but 47 is not divisible by 3, because the sum of its digits is 
4 + 7 = 11 that is not multiple of 3, and 11 is not divisible of 3.  
So, the original number is divisible by 3, if and only if the sum of its digits is divisible by 3.

Example 4: Is the number 222 divisible by 3.
Solution: Let’s add the digits of the number 222. 

We get 2 + 2 + 2 = 6, since the sum of the digits is 
divisible by 3.

Therefore, the number 222 is also divisible by 3.

If a number is a multiplication of 3 consecutive numbers then that number is always divisible by 3.

Example 4: Is the number 504 is divisible by 3.

Solution: 504 is multiplication of the numbers  

                       7 x 8 x 9 =  504

              ∴  original number is 504

                          504/3 = 168

We see that 789 is completely divided by 3.  

Divisibility Rules – Number Divisible by 4

A number is divisible by 4, if the last two digits of the number are divisible by 4.

Example 1: Is the number 624 is divisible by 4.

Solution: First we observe the last two digits of the given number, we notice that last two digits of the number is 24, and 24 is divisible by 4. So we conclude that 624 is divisible by 4. 

Example 2: In the set of numbers 715, 413, 948, 508, and 618, find the numbers which are divisible by 4.

Solution: We observe the last two digits of the given numbers.

1. Last two digits of the number 715 is 15, since 15 is not divisible by 4, so 715 is not divisible by 4. 

2. Last two digits of the number 413 is 13, since 13 is not divisible by 4, so 413 is not divisible by 4. 

3. Last two digits of the number 948 is 48, since 48 is divisible by 4, so 948 is divisible by 4.

4. Last two digits of the number 508 is 08, since 08 is divisible by 4, so 508 is divisible by 4.

5. Last two digits of the number 618 is 18, since 18 is not divisible by 4, so 618 is not divisible by 4.

Example 3: Number 612 has last two digits 12, since 12 is divisible by 4, so 612 is divisible by 4.

Example 4: Number 216 has last two digits 16, since 16 is divisible by 4, so 216 is divisible by 4.

Example 5: Number 2068 has last two digits 68, since 68 is divisible by 4, so 2068 is divisible by 4.

Example 6: Number 5789 has last two digits 89, since 89 is not divisible by 4, so 5789 is not divisible by 4. 

Divisibility Rules – Number Divisible by 5

A number is divisible by 5, if the last two digits of the number must be 0 or 5.

Example:

1. 20 is divisible by 5 as last digit of 20 is 0.

2. 40 is divisible by 5 as last digit of 40 is 0. 

3. 150 is divisible by 5 as last digit of 150 is 0. 

4. 45 is divisible by 5 as last digit of 45 is 5. 

5. 165 is divisible by 5 as last digit of 165 is 5. 

6. 2475 is divisible by 5 as last digit of 2475 is 5. 

7. 37 is not divisible by 5 as last digit of 37 is not 0 or 5.

 8. 2963 is not divisible by 5 as last digit of 2963 is not 0 or 5.  

Divisibility Rules – Number Divisible by 6

A number is divisible by 6, if the number is divisible by both 2 and 3.

Example 1: 24 is divisible by 2, as last digit is 4 and sum of 24 is 6, which is divisible by 3. So 24 is divisible by 6.

Example 2: 54 is divisible by 2, as last digit is 4 and sum of 54 is 9, which is divisible by 3. So 54 is divisible by 6.

Example 3: 108 is divisible by 2, as last digit is 8 and sum of 108 is 9, which is divisible by 3. So 108 is divisible by 6.

Example 4: 144 is divisible by 2, because last digit of the number is is 4 and sum of 144 is 9, which is divisible by 3. 144 ÷ 6 = 24, So, 144 is divisible by 6.

Example 5: 355 is not divisible by 2, because last digit of 355 is 5, means it is not divisible by 2. 

If a number divisible by 6, it must be divisible by 2 and 3. So there is no need to check further and we conclude that the number is not divisible by 6. 

Example 6: We check the number 5106 is divisible by 6 or not. 

First we check the last digit of the given number that is 6, so 6 is divisible by 2. 

Now we check the number 5106 is divisible by 3. 

The sum of digits of the number 5106 is 12, obviously the sum of digits is divisible by 3, 12 ÷ 3 = 4. 

Since the number 5106 is divisible by both 2 and 3.

 Therefore, the number is divisible by 6. 

Divisibility Rules – Number Divisible by 7

If we subtract twice of last digit from the number, formed by remaining digits is divisible by 7, then the number is divisible by 7.

Example 1:  We check the number 546 is divisible by 7 or not.

Solution: Last digit of the number 546 is 6, it’s twice is 6 x 2 = 12.

Now we subtract 12 from remaining number 54.

 54 – 12 = 42. 42 is divisible by 7,

since 546 is divisible by 7.

Example 2: We check the number 805 is divisible by 7 or not.

Solution: Last digit of the number 805 is 5, it’s twice is 5 x 2 = 10.

Now we subtract 10 from remaining number 80.     

80 – 10 = 70.

70 is divisible by 7,

70 ÷ 7 = 10, 

since 805 is divisible by 7.

Example 3: Is the number 6013 is divisible by 7.

Solution: Last digit of number the 6013 is 3, it’s twice is 3 x 2 = 6.

Now we subtract 6 from remaining number 601.     

601 – 6 = 595.

595 is divisible by 7,

595 ÷ 7 = 85, since 546 is divisible by 7.

Example 4: Is the number 972 is divisible by 7.

Solution: Last digit of the number 972 is 2, it’s twice is 2 x 2 = 4.

Now we subtract 4 from remaining number 97.     

97 – 4 = 93. 93 is not divisible by 7, 93 ÷ 7 = 13.2, 

since 972 is not divisible by 7.

Example 5: Is the number 4215 is divisible by 7.

Solution: Last digit of the number 4215 is 5, it’s twice is 5 x 2 = 10.

Now we subtract 10 from remaining number 421. 

421 – 10 = 411.

411 is not divisible by 7,

411 ÷ 7 = 58.7, 

since 4215 is not divisible by 7.

Divisibility Rules – Number Divisible by 8

If the last three digits of a whole number are divisible by 8, then the number is divisible by 8.

Example 1: Is the number 4784 is divisible by 8.

Solution: Using the test of divisibility rule for 8,

we look that the last three digits of the number, is 784.

784 is divisible by 8.

784 ÷ 8 = 98.

Since the number 4784 is divisible by 8.

Example 2: Is the number 4800 is divisible by 8.

Solution: Using the test of divisibility, we look that the last three digits of the number, is 800. 

800 is divisible by 8. 

800 ÷ 8 = 80.

Since the number 4800 is divisible by 8.

Example 3: Is the number 57968 is divisible by 8.

Solution: Using the test of divisibility, we look that the last three digits of the number, is 968.

 968 is divisible by 8.

968 ÷ 8 = 121.

Since the number 57968 is divisible by 8.

Example 4: Is the number 9521367 is divisible by 8.

Solution:Using the test of divisibility rule, we look that the last three digits of the number, is 367. 

367 is not divisible by 8.

9521367 ÷ 8 = 1190170.8.

Since the number 9521367 is not divisible by 8.

Divisibility Rules – Number Divisible by 9

A number divisible by 9, if the sum of digits is divisible by 9.

Example 1: Is the number 4536 is divisible by 9.

Solution: Using the test of divisibility rule for 9, we look that sum of the given numbers is 4 + 5 + 3 + 6 = 18.

18 is divisible by 9.

18 ÷ 9 = 2. 

So, the number 4536 is divisible by 9.

Example 2: Is the number 9468 is divisible by 9.

Solution: Using the test of divisibility rule for 9, we look that sum of the given numbers is 9 + 4 + 6 + 8 = 27.

27 is divisible by 9. 27 ÷ 9 = 3. 

So, the number 9468 is divisible by 9.

Example 3: Is the number 7532 is divisible by 9.

Solution: Using the test of divisibility rule for 9, we look that sum of the given numbers is 7 + 5 + 3 + 2 = 17.

17 is not divisible by 9.

17 ÷ 9 = 1.8 

So, the number 7532 is not divisible by 9.

Divisibility Rules – Number Divisible by 10

A number divisible by 10, if last digit of the number is 0.

A number divisible by 10, If it has 0 (zero) in its, ones place or if last digit of the number is 0.

The multiples of 10 is 10, 20, 30, 40, 50, 60 70….
We get something common in these numbers.

We see that last digit of each of these numbers is 0, or each number has 0 in its ones place.

Some more numbers like 100, 200, 1100, 1000, 420  4080, 5010, has 0 in ones place.

All numbers that has 0 in ones, place are divisible by 10.

So we find that A number divisible by 10, If it has 0 (zero) in the ones place.      

Divisibility Rules – Number Divisible by 11

If the difference of the sum of alternative digits of a number is divisible by 11, then the number is divisible by 11,

or

Sum of odd numbers – Sum of even numbers = 0 or 11. 

Example 1: To check the number 308 is divisible by 11, procedure is following.

Solution: Group the alternative digits i.e. digits which are in odd places together and digits in even places together. Here 38 and 0 are two groups.

Take the sum of the digits of each group i.e. 3 + 8 = 11 and 0 = 0.
Now find the difference of the sums 11 – 0 = 11

If the difference is divisible by 11, then the original number is also divisible by 11.

Here, 11 is the difference which is divisible by 11.Therefore, 308 is divisible by 11.

Example 2: To check the number 3154 is divisible by 11, procedure is following.

Solution: Group the alternative digits i.e. digits which are in odd places together and digits in even places together.

Here 35 and 14 are two groups.

Take the sum of the digits of each group i.e. 3 + 5 = 8 and 1 + 4 = 5.

Sum of odd numbers – Sum of even numbers = 0 or 11.

Now find the difference of the sums 8 – 5 = 3.

If the difference is divisible by 11, then the original number is also divisible by 11.

Here, 3 is the difference which is not divisible by 11.Therefore, 3145 is not divisible by 11.

Divisibility Rules – Number Divisible by 12

A number is divisible by 12, if the number is of divisible by both 4 and 3.

Example 1: Is the number 2148 is divisible by 12.

Solution: Number 2148, has sum of digits is 15, which is divisible by 3. So the number is divisible by 3.

2148 has last two digits 48, and 48 is divisible by 4. So, 2148 is divisible by 4.

Therefore, the number 2148 is divisible by both 3 and 4, it is also divisible by 12.

Example 2: Is the number 1140 is divisible by 12.

Solution: Number 1140, has sum of digits is 6, which is divisible by 3. So the number is divisible by 3.

1140 has last two digits 40, and 40 is divisible by 4. So 1140 is divisible by 4.

Therefore, the number 1140 is divisible by both 3 and 4, it is also divisible by 12.

Example 3: Is the number 7896 is divisible by 12.

Solution: Number 7896, has sum of digits is 30, which is divisible by 3. So the number is divisible by 3.

7896 has last two digits 96, and 96 is divisible by 4. So 7896 is divisible by 4.

Therefore, the number 7896 is divisible by both 3 and 4, it is also divisible by 12

Example 4: Is the number 8973 is divisible by 12.

Solution: Number 8971, has sum of digits is 25, which is not divisible by 3.

So the number is not divisible by 3.

8971 has last two digits 71, and 71 is not divisible by 4. So 8971 is not divisible by 4.

Therefore, the number 8971 is not divisible by both 3 and 4. It is not divisible by 12

Example 5: Is the number 5425 is divisible by 12.

Solution: Number 5425, has sum of digits is 16, which is not divisible by 3.

So the number is not divisible by 3.

5425 has last two digits 25, and 25 is not divisible by 4.

 So 5425 is not divisible by 4.

Therefore, the number 5425 is not divisible by both 3 and 4. It is not divisible by 12.

Divisibility Rules – Number Divisible by 13

A number is divisible by 13, if we have to add four times of the last digit number, to the remaining number and repeat the process until we get a two-digit number.

To check the divisibility of 13, in any given number,
we have to add four times of the last digit number to the remaining number and repeat the process until we get a two-digit number.

Now check if that two-digit number is divisible by 13 or not. if it is divisible then the given number is divisible by 13.

Example 1: 169 → 16 + (9 x 4)→ 52. Number 52 is divisible by 13, so 169 is divisible by 13.

Example 2: 1287 → 128 + (7 x 4)→ 128 + 28 → 
156 → 15 + (6 x 4) → 15 + 24 → 39.

Number 39 is divisible by 13, so 1287 is divisible by 13.

Example 3: 1612 → 161 + (2 x 4)→ 161 + 8 → 

169 → 16 + (9 x 4) → 16 + 36 → 52.

Number 52 is divisible by 13, so 1612 is divisible by 13.

Example 4: 169 → 16 + (9 x 4)→ 52.

 Number 52 is divisible by 13, so 169 is divisible by 13.

Example 5: 1725 → 172 + (5 x 4)→ 172 + 20 → 
192 → 19 + (2 x 4) → 19 + 8 → 27.

Number 27 is not divisible by 13, so 1725 is not divisible by 13.

Example 5: 1934 → 193 + (4 x 4)→ 193 + 16 → 

209 → 20 + (9 x 4) → 20 + 36 → 56.

Number 56 is not divisible by 13, so 1934 is not divisible by 13.

Divisibility Rules – Number Divisible by 14

Add the last two digits, to twice the rest. The result must be divisible by 14.

Example 1: Is the number 1064 is divisible by 14.

1064 → (10 x 2) + 64 = 84.

84 is divisible by 14. 84 ÷ 14 = 6.

532 → (5 x 2) + 32 = 42. 42 is divisible by 14.

42 ÷ 14 = 3.

1372 → (13 x 2) + 72 = 98.

98 is divisible by 14. 98 ÷ 14 = 7.

9267 → (92 x 2) + 67 = 251. 251 is not divisible by 14. 

5327 → (53 x 2) + 27 = 133. 133 is divisible by 14.